Article contents
On the cubic NLS on 3D compact domains
Published online by Cambridge University Press: 04 February 2013
Abstract
We prove bilinear estimates for the Schrödinger equation on 3D domains, with Dirichlet boundary conditions. On non-trapping domains, they match the  ${ \mathbb{R} }^{3} $ case, while on bounded domains they match the generic boundaryless manifold case. We obtain, as an application, global well-posedness for the defocusing cubic NLS for data in
${ \mathbb{R} }^{3} $ case, while on bounded domains they match the generic boundaryless manifold case. We obtain, as an application, global well-posedness for the defocusing cubic NLS for data in 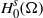 ${ H}_{0}^{s} (\Omega )$,
${ H}_{0}^{s} (\Omega )$,  $1\lt s\leq 3$, with
$1\lt s\leq 3$, with 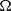 $\Omega $ any bounded domain with smooth boundary.
$\Omega $ any bounded domain with smooth boundary.
Résumé
On démontre des estimations bilinéaires pour l’équation de Schrödinger sur des domaines tridimensionnels, avec condition de Dirichlet au bord. Dans le cas non-captant, on retrouve les estimations connues dans  ${ \mathbb{R} }^{3} $, et sur un domaine borné on obtient des estimations similaires à celles du cas d’une variété compacte générique sans bord. Une application est donnée à l’existence de solutions globales dans
${ \mathbb{R} }^{3} $, et sur un domaine borné on obtient des estimations similaires à celles du cas d’une variété compacte générique sans bord. Une application est donnée à l’existence de solutions globales dans 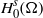 ${ H}_{0}^{s} (\Omega )$,
${ H}_{0}^{s} (\Omega )$, 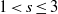 $1\lt s\leq 3$, avec
$1\lt s\leq 3$, avec  $\Omega $ un domaine borné régulier.
$\Omega $ un domaine borné régulier.
Keywords
Information
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 13 , Issue 1 , January 2014 , pp. 1 - 18
- Copyright
- ©Cambridge University Press 2013
References
- 2
- Cited by

