Published online by Cambridge University Press: 15 May 2020
Let  $F$ be a totally real field in which
$F$ be a totally real field in which  $p$ is unramified. Let
$p$ is unramified. Let  $\overline{r}:G_{F}\rightarrow \text{GL}_{2}(\overline{\mathbf{F}}_{p})$ be a modular Galois representation that satisfies the Taylor–Wiles hypotheses and is tamely ramified and generic at a place
$\overline{r}:G_{F}\rightarrow \text{GL}_{2}(\overline{\mathbf{F}}_{p})$ be a modular Galois representation that satisfies the Taylor–Wiles hypotheses and is tamely ramified and generic at a place  $v$ above
$v$ above 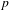 $p$. Let
$p$. Let 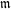 $\mathfrak{m}$ be the corresponding Hecke eigensystem. We describe the
$\mathfrak{m}$ be the corresponding Hecke eigensystem. We describe the 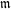 $\mathfrak{m}$-torsion in the
$\mathfrak{m}$-torsion in the 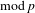 $\text{mod}\,p$ cohomology of Shimura curves with full congruence level at
$\text{mod}\,p$ cohomology of Shimura curves with full congruence level at  $v$ as a
$v$ as a 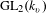 $\text{GL}_{2}(k_{v})$-representation. In particular, it only depends on
$\text{GL}_{2}(k_{v})$-representation. In particular, it only depends on 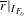 $\overline{r}|_{I_{F_{v}}}$ and its Jordan–Hölder factors appear with multiplicity one. The main ingredients are a description of the submodule structure for generic
$\overline{r}|_{I_{F_{v}}}$ and its Jordan–Hölder factors appear with multiplicity one. The main ingredients are a description of the submodule structure for generic  $\text{GL}_{2}(\mathbf{F}_{q})$-projective envelopes and the multiplicity one results of Emerton, Gee and Savitt [Lattices in the cohomology of Shimura curves, Invent. Math. 200(1) (2015), 1–96].
$\text{GL}_{2}(\mathbf{F}_{q})$-projective envelopes and the multiplicity one results of Emerton, Gee and Savitt [Lattices in the cohomology of Shimura curves, Invent. Math. 200(1) (2015), 1–96].