Article contents
Des points fixes communs pour des difféomorphismes de 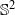 ${ \mathbb{S} }^{2} $ qui commutent et préservent une mesure de probabilité
${ \mathbb{S} }^{2} $ qui commutent et préservent une mesure de probabilité
Published online by Cambridge University Press: 08 February 2013
Résumé
Nous montrons des résultats d’existence de points fixes communs pour des homéomorphismes du plan 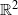 ${ \mathbb{R} }^{2} $ ou la sphère
${ \mathbb{R} }^{2} $ ou la sphère 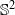 ${ \mathbb{S} }^{2} $, qui commutent deux à deux et préservent une mesure de probabilité. Par exemple, nous montrons que des
${ \mathbb{S} }^{2} $, qui commutent deux à deux et préservent une mesure de probabilité. Par exemple, nous montrons que des  ${C}^{1} $-difféomorphismes
${C}^{1} $-difféomorphismes 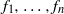 ${f}_{1} , \ldots , {f}_{n} $ de
${f}_{1} , \ldots , {f}_{n} $ de 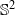 ${ \mathbb{S} }^{2} $ suffisamment proches de l’identité, qui commutent deux à deux, et qui préservent une mesure de probabilité dont le support n’est pas réduit à un point, ont au moins deux points fixes communs.
${ \mathbb{S} }^{2} $ suffisamment proches de l’identité, qui commutent deux à deux, et qui préservent une mesure de probabilité dont le support n’est pas réduit à un point, ont au moins deux points fixes communs.
Abstract
We prove the existence of common fixed points for some homeomorphisms of the plane 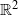 ${ \mathbb{R} }^{2} $ or the two-sphere
${ \mathbb{R} }^{2} $ or the two-sphere 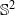 ${ \mathbb{S} }^{2} $ which commute and preserve a probability measure. For example, if
${ \mathbb{S} }^{2} $ which commute and preserve a probability measure. For example, if 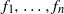 ${f}_{1} , \ldots , {f}_{n} $ are commuting
${f}_{1} , \ldots , {f}_{n} $ are commuting 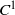 ${C}^{1} $-diffeomorphisms of
${C}^{1} $-diffeomorphisms of 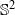 ${ \mathbb{S} }^{2} $ that are sufficiently close to the identity, and that preserve a probability measure whose support is not a single point, then they have at least two common fixed points.
${ \mathbb{S} }^{2} $ that are sufficiently close to the identity, and that preserve a probability measure whose support is not a single point, then they have at least two common fixed points.
Keywords
MSC classification
Information
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 12 , Issue 4 , October 2013 , pp. 821 - 851
- Copyright
- ©Cambridge University Press 2013
References
Bibliographie
- 2
- Cited by

