Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
GEE, TOBY
and
KISIN, MARK
2014.
THE BREUIL–MÉZARD CONJECTURE FOR POTENTIALLY
BARSOTTI–TATE REPRESENTATIONS.
Forum of Mathematics, Pi,
Vol. 2,
Issue. ,
Paškūnas, Vytautas
2015.
On the Breuil–Mézard conjecture.
Duke Mathematical Journal,
Vol. 164,
Issue. 2,
Cheng, Chuangxun
2015.
Explicit examples for the Breuil–Mézard conjecture.
Journal of Number Theory,
Vol. 157,
Issue. ,
p.
199.
Emerton, Matthew
Gee, Toby
and
Savitt, David
2015.
Lattices in the cohomology of Shimura curves.
Inventiones mathematicae,
Vol. 200,
Issue. 1,
p.
1.
Shotton, Jack
2016.
Local deformation rings for GL2 and a Breuil–Mézard conjecture when l≠p.
Algebra & Number Theory,
Vol. 10,
Issue. 7,
p.
1437.
Hellmann, Eugen
and
Schraen, Benjamin
2016.
Density of potentially crystalline representations of fixed weight.
Compositio Mathematica,
Vol. 152,
Issue. 8,
p.
1609.
Park, Chol
2016.
Regular filtered (ϕ,N)-modules of dimension 3.
Journal of Number Theory,
Vol. 163,
Issue. ,
p.
406.
Paškūnas, Vytautas
2016.
On 2-dimensional 2-adic Galois representations of local and global fields.
Algebra & Number Theory,
Vol. 10,
Issue. 6,
p.
1301.
Park, Chol
2017.
Reduction modulo 𝑝 of certain semi-stable representations.
Transactions of the American Mathematical Society,
Vol. 369,
Issue. 8,
p.
5425.
Breuil, Christophe
Hellmann, Eugen
and
Schraen, Benjamin
2017.
Une interprétation modulaire de la variété trianguline.
Mathematische Annalen,
Vol. 367,
Issue. 3-4,
p.
1587.
Nadimpalli, Santosh
2017.
Typical representations for level zero Bernstein components of GL (F).
Journal of Algebra,
Vol. 469,
Issue. ,
p.
1.
Paškūnas, Vytautas
2017.
On 2-adic deformations.
Mathematische Zeitschrift,
Vol. 286,
Issue. 3-4,
p.
801.
Herzig, Florian
Le, Daniel
and
Morra, Stefano
2017.
On mod local-global compatibility for in the ordinary case.
Compositio Mathematica,
Vol. 153,
Issue. 11,
p.
2215.
Le, Daniel
Le Hung, Bao V.
Levin, Brandon
and
Morra, Stefano
2018.
Potentially crystalline deformation rings and Serre weight conjectures: shapes and shadows.
Inventiones mathematicae,
Vol. 212,
Issue. 1,
p.
1.
Le, Daniel
2018.
Lattices in the cohomology of U(3) arithmetic manifolds.
Mathematische Annalen,
Vol. 372,
Issue. 1-2,
p.
55.
Le, Daniel
Morra, Stefano
and
Park, Chol
2018.
On mod p local-global compatibility for GL3(Qp) in the non-ordinary case.
Proceedings of the London Mathematical Society,
Vol. 117,
Issue. 4,
p.
790.
Caruso, Xavier
David, Agnès
and
Mézard, Ariane
2018.
VARIÉTÉS DE KISIN STRATIFIÉES ET DÉFORMATIONS POTENTIELLEMENT BARSOTTI-TATE.
Journal of the Institute of Mathematics of Jussieu,
Vol. 17,
Issue. 5,
p.
1019.
Shotton, Jack
2018.
The Breuil–Mézard conjecture when l≠p.
Duke Mathematical Journal,
Vol. 167,
Issue. 4,
Hu, Yongquan
and
Paškūnas, Vytautas
2019.
On crystabelline deformation rings of $$\mathrm {Gal}(\overline{\mathbb {Q}}_p/\mathbb {Q}_p)$$ Gal ( Q ¯ p / Q p ) (with an appendix by Jack Shotton).
Mathematische Annalen,
Vol. 373,
Issue. 1-2,
p.
421.
Nadimpalli, Santosh
2019.
On classification of typical representations for GL3(F).
Forum Mathematicum,
Vol. 31,
Issue. 4,
p.
917.
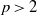 $p\gt 2$ be prime. We state and prove (under mild hypotheses on the residual representation) a geometric refinement of the Breuil–Mézard conjecture for two-dimensional mod
$p\gt 2$ be prime. We state and prove (under mild hypotheses on the residual representation) a geometric refinement of the Breuil–Mézard conjecture for two-dimensional mod 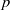 $p$ representations of the absolute Galois group of
$p$ representations of the absolute Galois group of 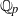 ${ \mathbb{Q} }_{p} $. We also state a conjectural generalization to
${ \mathbb{Q} }_{p} $. We also state a conjectural generalization to  $n$-dimensional representations of the absolute Galois group of an arbitrary finite extension of
$n$-dimensional representations of the absolute Galois group of an arbitrary finite extension of 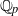 ${ \mathbb{Q} }_{p} $, and give a conditional proof of this conjecture, subject to a certain
${ \mathbb{Q} }_{p} $, and give a conditional proof of this conjecture, subject to a certain  $R= \mathbb{T} $-type theorem together with a strong version of the weight part of Serre’s conjecture for rank
$R= \mathbb{T} $-type theorem together with a strong version of the weight part of Serre’s conjecture for rank  $n$ unitary groups. We deduce an unconditional result in the case of two-dimensional potentially Barsotti–Tate representations.
$n$ unitary groups. We deduce an unconditional result in the case of two-dimensional potentially Barsotti–Tate representations.
