Article contents
Extensions of differential representations of SL2 and tori
Published online by Cambridge University Press: 16 May 2012
Abstract
Linear differential algebraic groups (LDAGs) measure differential algebraic dependencies among solutions of linear differential and difference equations with parameters, for which LDAGs are Galois groups. Differential representation theory is a key to developing algorithms computing these groups. In the rational representation theory of algebraic groups, one starts with 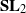 and tori to develop the rest of the theory. In this paper, we give an explicit description of differential representations of tori and differential extensions of irreducible representation of
and tori to develop the rest of the theory. In this paper, we give an explicit description of differential representations of tori and differential extensions of irreducible representation of 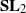 . In these extensions, the two irreducible representations can be non-isomorphic. This is in contrast to differential representations of tori, which turn out to be direct sums of isotypic representations.
. In these extensions, the two irreducible representations can be non-isomorphic. This is in contrast to differential representations of tori, which turn out to be direct sums of isotypic representations.
Keywords
Information
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 12 , Issue 1 , January 2013 , pp. 199 - 224
- Copyright
- Copyright © Cambridge University Press 2013
References
- 8
- Cited by

