Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kublanovsky, S. I.
Lee, Edmond W. H.
and
Reilly, Norman R.
2008.
Some conditions related to the exactness of Rees-Sushkevich varieties.
Semigroup Forum,
Vol. 76,
Issue. 1,
p.
87.
KELAREV, A. V.
WATTERS, P.
and
YEARWOOD, J. L.
2009.
REES MATRIX CONSTRUCTIONS FOR CLUSTERING OF DATA.
Journal of the Australian Mathematical Society,
Vol. 87,
Issue. 3,
p.
377.
Kelarev, A. V.
Yearwood, J. L.
Watters, P.
Wu, X.
Abawajy, J. H.
and
Pan, L.
2010.
Internet security applications of the Munn rings.
Semigroup Forum,
Vol. 81,
Issue. 1,
p.
162.
Reilly, Norman R.
2012.
Bipartite graphs and completely 0-simple semigroups.
Semigroup Forum,
Vol. 84,
Issue. 1,
p.
176.
JONES, PETER R.
2013.
THE SEMIGROUPS B2AND B0ARE INHERENTLY NONFINITELY BASED, AS RESTRICTION SEMIGROUPS.
International Journal of Algebra and Computation,
Vol. 23,
Issue. 06,
p.
1289.
Reilly, Norman R.
2013.
Regular principal factors in free objects in Rees-Sushkevich varieties.
Semigroup Forum,
Vol. 86,
Issue. 1,
p.
162.
Reilly, Norman R.
2014.
Regular principal factors in free objects in varieties in the interval [B 2 ,NB 2 ∨G n ].
Semigroup Forum,
Vol. 89,
Issue. 1,
p.
249.
Lee, Edmond W. H.
2023.
Advances in the Theory of Varieties of Semigroups.
p.
1.

 (iii)
(iii) 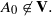 The conditions (i) and (ii) are also sufficient conditions. In this note, we complete Kublanovsky’s programme by refining condition (iii) to obtain a complete set of conditions that are both necessary and sufficient.
The conditions (i) and (ii) are also sufficient conditions. In this note, we complete Kublanovsky’s programme by refining condition (iii) to obtain a complete set of conditions that are both necessary and sufficient.