No CrossRef data available.
Published online by Cambridge University Press: 07 June 2013
An unknotting tunnel in a 3-manifold with boundary is a properly embedded arc, the complement of an open neighborhood of which is a handlebody. A geodesic with endpoints on the cusp boundary of a hyperbolic 3-manifold and perpendicular to the cusp boundary is called a vertical geodesic. Given a vertical geodesic  $\alpha $ in a hyperbolic 3-manifold
$\alpha $ in a hyperbolic 3-manifold 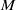 $M$, we find sufficient conditions for it to be an unknotting tunnel. In particular, if
$M$, we find sufficient conditions for it to be an unknotting tunnel. In particular, if  $\alpha $ corresponds to a 4-bracelet, 5-bracelet or 6-bracelet in the universal cover and has short enough length, it must be an unknotting tunnel. Furthermore, we consider a vertical geodesic
$\alpha $ corresponds to a 4-bracelet, 5-bracelet or 6-bracelet in the universal cover and has short enough length, it must be an unknotting tunnel. Furthermore, we consider a vertical geodesic  $\alpha $ that satisfies the elder sibling property, which means that in the universal cover, every horoball except the one centered at
$\alpha $ that satisfies the elder sibling property, which means that in the universal cover, every horoball except the one centered at 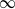 $\infty $ is connected to a larger horoball by a lift of
$\infty $ is connected to a larger horoball by a lift of  $\alpha $. Such an
$\alpha $. Such an  $\alpha $ with length less than
$\alpha $ with length less than 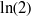 $\ln (2)$ is then shown to be an unknotting tunnel.
$\ln (2)$ is then shown to be an unknotting tunnel.