Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Nakamoto, Kazunori
2014.
The moduli of representations with Borel mold.
International Journal of Mathematics,
Vol. 25,
Issue. 07,
p.
1450067.
Banica, Teodor
and
Chirvasitu, Alexandru
2019.
Quasi-flat representations of uniform groups and quantum groups.
Journal of Algebra and Its Applications,
Vol. 18,
Issue. 08,
p.
1950155.

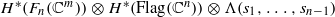 are isomorphic as algebras. Here the degree of
are isomorphic as algebras. Here the degree of 