No CrossRef data available.
Published online by Cambridge University Press: 01 May 2013
We investigate island systems with continuous height functions and strongly laminar systems which are laminar systems containing sets with disjoint boundaries. In the discrete case, we show that for a maximal rectangular system of islands 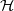 $ \mathcal{H} $ on an
$ \mathcal{H} $ on an 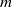 $m$ by
$m$ by  $n$ rectangular grid we have
$n$ rectangular grid we have 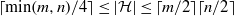 $\lceil \min (m, n)/ 4\rceil \leq \vert \mathcal{H} \vert \leq \lceil m/ 2\rceil \lceil n/ 2\rceil $. In the continuous case we show that under some conditions maximal strongly laminar systems
$\lceil \min (m, n)/ 4\rceil \leq \vert \mathcal{H} \vert \leq \lceil m/ 2\rceil \lceil n/ 2\rceil $. In the continuous case we show that under some conditions maximal strongly laminar systems  $ \mathcal{H} $ have cardinality
$ \mathcal{H} $ have cardinality 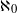 ${\aleph }_{0} $ or
${\aleph }_{0} $ or 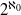 ${2}^{{\aleph }_{0} } $ and present examples with
${2}^{{\aleph }_{0} } $ and present examples with 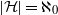 $\vert \mathcal{H} \vert = {\aleph }_{0} $.
$\vert \mathcal{H} \vert = {\aleph }_{0} $.