Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Nikmehr, M. J.
and
Fatahi, F.
2011.
Remarks on α-strongly irreducible ideals.
Frontiers of Mathematics in China,
Vol. 6,
Issue. 5,
p.
901.
Juett, Jason
2012.
Generalized comaximal factorization of ideals.
Journal of Algebra,
Vol. 352,
Issue. 1,
p.
141.
Abbasi, A.
and
Hassanzadeh-lelekaami, D.
2012.
Quasi-prime Submodules and Developed Zariski Topology.
Algebra Colloquium,
Vol. 19,
Issue. spec01,
p.
1089.
ABUHLAIL, JAWAD
and
LOMP, CHRISTIAN
2013.
ON THE NOTION OF STRONG IRREDUCIBILITY AND ITS DUAL.
Journal of Algebra and Its Applications,
Vol. 12,
Issue. 06,
p.
1350012.
Nikseresht, A.
and
Azizi, A.
2013.
Zariski spaces of modules.
Journal of Pure and Applied Algebra,
Vol. 217,
Issue. 7,
p.
1187.
Nikseresht, A.
and
Azizi, A.
2014.
Semi-irreducible Zariski spaces of modules.
Journal of Algebra and Its Applications,
Vol. 13,
Issue. 08,
p.
1450054.
Schwartz, Niels
2016.
Strongly Irreducible Ideals and Truncated Valuations.
Communications in Algebra,
Vol. 44,
Issue. 3,
p.
1055.
Hassanzadeh-lelekaami, D.
2020.
On the Prime Spectrum of Torsion Modules.
Iranian Journal of Mathematical Sciences and Informatics,
Vol. 15,
Issue. 1,
p.
53.
Zeidi, Nabil
2020.
On n-irreducible ideals of commutative rings.
Journal of Algebra and Its Applications,
Vol. 19,
Issue. 06,
p.
2050120.
Çeken, Seçil
and
Yüksel, Cem
2021.
Generalizations of strongly hollow ideals and a corresponding topology.
AIMS Mathematics,
Vol. 6,
Issue. 12,
p.
12986.
Rostami, Esmaeil
2021.
Strongly hollow elements of commutative rings.
Journal of Algebra and Its Applications,
Vol. 20,
Issue. 06,
p.
2150107.
Hassanzadeh-lelekaami, Dawood
2022.
A generalization of Hartshorne's connectedness theorem.
Boletim da Sociedade Paranaense de Matemática,
Vol. 40,
Issue. ,
p.
1.
Abuhlail, Jawad
and
Hroub, Hamza
2022.
PS-hollow representations of modules over commutative rings.
Journal of Algebra and Its Applications,
Vol. 21,
Issue. 02,
Darani, Ahmad Yousefian
Mahdou, Najib
and
Moussaoui, Sanae
2022.
Strongly ϕ-n-irreducible ideals.
Journal of Algebra and Its Applications,
Vol. 21,
Issue. 12,
Goswami, A.
2023.
Normal structure spaces of groups.
Rendiconti del Circolo Matematico di Palermo Series 2,
Vol. 72,
Issue. 7,
p.
3697.
Goswami, A.
2023.
Iséki spaces of semirings.
Bollettino dell'Unione Matematica Italiana,
Vol. 16,
Issue. 4,
p.
677.
Dube, Themba
and
Goswami, Amartya
2023.
Ideal spaces: An extension of structure spaces of rings.
Journal of Algebra and Its Applications,
Vol. 22,
Issue. 11,
Hashemi, Jamal
and
Yari, Hossein
2024.
A generalization of strongly irreducible ideals with a view towards rings of continuous functions.
Applied General Topology,
Vol. 25,
Issue. 2,
p.
491.
Goswami, Amartya
and
Dube, Themba
2024.
Some aspects of k-ideals of semirings.
Rendiconti del Circolo Matematico di Palermo Series 2,
Vol. 73,
Issue. 8,
p.
3105.
Goswami, Amartya
2025.
Some topological aspects of ideals in quantales.
Applied General Topology,
Vol. 26,
Issue. 1,
p.
483.
 implies that either
implies that either 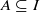 or
or  . In this paper we study strongly irreducible ideals in different rings. The relations between strongly irreducible ideals of a ring and strongly irreducible ideals of localizations of the ring are also studied. Furthermore, a topology similar to the Zariski topology related to strongly irreducible ideals is introduced. This topology has the Zariski topology defined by prime ideals as one of its subspace topologies.
. In this paper we study strongly irreducible ideals in different rings. The relations between strongly irreducible ideals of a ring and strongly irreducible ideals of localizations of the ring are also studied. Furthermore, a topology similar to the Zariski topology related to strongly irreducible ideals is introduced. This topology has the Zariski topology defined by prime ideals as one of its subspace topologies.