Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Vural, Çetin
2008.
Paracompactness of paratopological groups which are GO-spaces.
Topology and its Applications,
Vol. 155,
Issue. 12,
p.
1329.

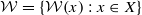 satisfies condition (
satisfies condition (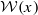 is of the form
is of the form 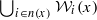 , where
, where  is Noetherian of finite rank, and every other
is Noetherian of finite rank, and every other 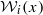 is a chain (with respect to inclusion) of neighbourhoods of
is a chain (with respect to inclusion) of neighbourhoods of  of point-finite rank, then
of point-finite rank, then 