Published online by Cambridge University Press: 08 January 2020
We determine, up to multiplicative constants, the number of integers 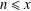 $n\leq x$ that have a divisor in
$n\leq x$ that have a divisor in 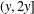 $(y,2y]$ and no prime factor
$(y,2y]$ and no prime factor  $\leq w$. Our estimate is uniform in
$\leq w$. Our estimate is uniform in 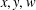 $x,y,w$. We apply this to determine the order of the number of distinct integers in the
$x,y,w$. We apply this to determine the order of the number of distinct integers in the 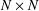 $N\times N$ multiplication table, which are free of prime factors
$N\times N$ multiplication table, which are free of prime factors 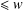 $\leq w$, and the number of distinct fractions of the form
$\leq w$, and the number of distinct fractions of the form 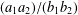 $(a_{1}a_{2})/(b_{1}b_{2})$ with
$(a_{1}a_{2})/(b_{1}b_{2})$ with  $1\leq a_{1}\leq b_{1}\leq N$ and
$1\leq a_{1}\leq b_{1}\leq N$ and 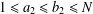 $1\leq a_{2}\leq b_{2}\leq N$.
$1\leq a_{2}\leq b_{2}\leq N$.
Communicated by I. Shparlinski
Research supported by National Science Foundation grant DMS-1802139.