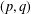 $(p,q)$-LAPLACIAN COUPLED SYSTEMS INVOLVING SCHRÖDINGER EQUATIONS
$(p,q)$-LAPLACIAN COUPLED SYSTEMS INVOLVING SCHRÖDINGER EQUATIONSPublished online by Cambridge University Press: 29 July 2019
We study the existence of positive ground state solutions for the following class of 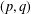 $(p,q)$-Laplacian coupled systems
$(p,q)$-Laplacian coupled systems 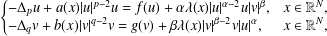 $$\begin{eqnarray}\left\{\begin{array}{@{}lr@{}}-\unicode[STIX]{x1D6E5}_{p}u+a(x)|u|^{p-2}u=f(u)+\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D706}(x)|u|^{\unicode[STIX]{x1D6FC}-2}u|v|^{\unicode[STIX]{x1D6FD}}, & x\in \mathbb{R}^{N},\\ -\unicode[STIX]{x1D6E5}_{q}v+b(x)|v|^{q-2}v=g(v)+\unicode[STIX]{x1D6FD}\unicode[STIX]{x1D706}(x)|v|^{\unicode[STIX]{x1D6FD}-2}v|u|^{\unicode[STIX]{x1D6FC}}, & x\in \mathbb{R}^{N},\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\left\{\begin{array}{@{}lr@{}}-\unicode[STIX]{x1D6E5}_{p}u+a(x)|u|^{p-2}u=f(u)+\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D706}(x)|u|^{\unicode[STIX]{x1D6FC}-2}u|v|^{\unicode[STIX]{x1D6FD}}, & x\in \mathbb{R}^{N},\\ -\unicode[STIX]{x1D6E5}_{q}v+b(x)|v|^{q-2}v=g(v)+\unicode[STIX]{x1D6FD}\unicode[STIX]{x1D706}(x)|v|^{\unicode[STIX]{x1D6FD}-2}v|u|^{\unicode[STIX]{x1D6FC}}, & x\in \mathbb{R}^{N},\end{array}\right.\end{eqnarray}$$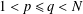 $1<p\leq q<N$. Here the coefficient
$1<p\leq q<N$. Here the coefficient 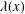 $\unicode[STIX]{x1D706}(x)$ of the coupling term is related to the potentials by the condition
$\unicode[STIX]{x1D706}(x)$ of the coupling term is related to the potentials by the condition 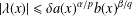 $|\unicode[STIX]{x1D706}(x)|\leq \unicode[STIX]{x1D6FF}a(x)^{\unicode[STIX]{x1D6FC}/p}b(x)^{\unicode[STIX]{x1D6FD}/q}$, where
$|\unicode[STIX]{x1D706}(x)|\leq \unicode[STIX]{x1D6FF}a(x)^{\unicode[STIX]{x1D6FC}/p}b(x)^{\unicode[STIX]{x1D6FD}/q}$, where 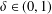 $\unicode[STIX]{x1D6FF}\in (0,1)$ and
$\unicode[STIX]{x1D6FF}\in (0,1)$ and 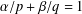 $\unicode[STIX]{x1D6FC}/p+\unicode[STIX]{x1D6FD}/q=1$. Using a variational approach based on minimization over the Nehari manifold, we establish the existence of positive ground state solutions for a large class of nonlinear terms and potentials.
$\unicode[STIX]{x1D6FC}/p+\unicode[STIX]{x1D6FD}/q=1$. Using a variational approach based on minimization over the Nehari manifold, we establish the existence of positive ground state solutions for a large class of nonlinear terms and potentials.
Research supported in part by INCTmat/MCT/Brazil, CNPq and CAPES/Brazil. The third author was also partially supported by Fapeg/CNpq grants 03/2015-PPP.