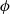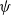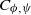Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Peng, Xiao-Feng
and
Jiang, Zhi-Jie
2024.
Order-Bounded Difference in Weighted Composition Operators Between Fock Spaces.
Mathematics,
Vol. 12,
Issue. 21,
p.
3360.
Mengestie, Tesfa
2024.
Surjective and closed range differentiation operator.
Rendiconti del Circolo Matematico di Palermo Series 2,
Vol. 73,
Issue. 5,
p.
1975.
Lo, Ching-on
and
Loh, Anthony Wai-keung
2025.
Stević-Sharma Type Operators on Bergman Spaces Induced by Doubling Weights.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 48,
Issue. 2,