No CrossRef data available.
Published online by Cambridge University Press: 09 April 2009
Let S(n) be a unilateral shift operator on a Hilbert space of multiplicity n. In this paper, we prove a generalization of the theorem that if S(1) is unitarily equivalent to an operator matrix form 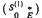 relative to a decomposition ℳ ⊕ N, then E is in a certain class C0 which will be defined below.
relative to a decomposition ℳ ⊕ N, then E is in a certain class C0 which will be defined below.