No CrossRef data available.
Published online by Cambridge University Press: 19 January 2015
Let  $S$ be a finitely generated pro-
$S$ be a finitely generated pro-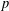 $p$ group. Let
$p$ group. Let 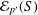 ${\mathcal{E}}_{p^{\prime }}(S)$ be the class of profinite groups
${\mathcal{E}}_{p^{\prime }}(S)$ be the class of profinite groups 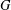 $G$ that have
$G$ that have 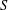 $S$ as a Sylow subgroup, and such that
$S$ as a Sylow subgroup, and such that 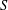 $S$ intersects nontrivially with every nontrivial normal subgroup of
$S$ intersects nontrivially with every nontrivial normal subgroup of 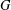 $G$. In this paper, we investigate whether or not there is a bound on
$G$. In this paper, we investigate whether or not there is a bound on 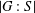 $|G:S|$ for
$|G:S|$ for  $G\in {\mathcal{E}}_{p^{\prime }}(S)$. For instance, we give an example where
$G\in {\mathcal{E}}_{p^{\prime }}(S)$. For instance, we give an example where 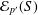 ${\mathcal{E}}_{p^{\prime }}(S)$ contains an infinite ascending chain of soluble groups, and on the other hand show that
${\mathcal{E}}_{p^{\prime }}(S)$ contains an infinite ascending chain of soluble groups, and on the other hand show that 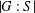 $|G:S|$ is bounded in the case where
$|G:S|$ is bounded in the case where 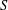 $S$ is just infinite.
$S$ is just infinite.