Published online by Cambridge University Press: 09 April 2009
We extend the normal surface Q-theory to non-compact 3-manifolds with respect to ideal triangulations. An ideal triangulation of a 3-manifold often has a small number of tetrahedra resulting in a system of Q-matching equations with a small number of variables. A unique feature of our approach is that a compact surface F with boundary properly embedded in a non-compact 3-manifold M with an ideal triangulation with torus cusps can be represented by a normal surface in M as follows. A half-open annulus made up of an infinite number of triangular disks is attached to each boundary component of F. The resulting surface 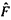 , when normalized, will contain only a finite number of Q-disks and thus correspond to an admissible solution to the system of Q-matching equations. The correspondence is bijective.
, when normalized, will contain only a finite number of Q-disks and thus correspond to an admissible solution to the system of Q-matching equations. The correspondence is bijective.