Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Whitney, Hassler
Birkhoff, Garrett
Mac Lane, Saunders
and
Higgs, D. A.
1986.
A Source Book in Matroid Theory.
p.
15.
Ardila, Federico
2003.
The Catalan matroid.
Journal of Combinatorial Theory, Series A,
Vol. 104,
Issue. 1,
p.
49.
Bonin, Joseph
de Mier, Anna
and
Noy, Marc
2003.
Lattice path matroids: enumerative aspects and Tutte polynomials.
Journal of Combinatorial Theory, Series A,
Vol. 104,
Issue. 1,
p.
63.
Crapo, Henry
and
Schmitt, William
2005.
A free subalgebra of the algebra of matroids.
European Journal of Combinatorics,
Vol. 26,
Issue. 7,
p.
1066.
Bonin, Joseph E.
and
de Mier, Anna
2006.
Lattice path matroids: Structural properties.
European Journal of Combinatorics,
Vol. 27,
Issue. 5,
p.
701.
Hine, Natalie
and
Oxley, James
2010.
When excluding one matroid prevents infinite antichains.
Advances in Applied Mathematics,
Vol. 45,
Issue. 1,
p.
74.
Bonin, Joseph E.
2010.
Lattice path matroids: The excluded minors.
Journal of Combinatorial Theory, Series B,
Vol. 100,
Issue. 6,
p.
585.
Hampe, Simon
2017.
The intersection ring of matroids.
Journal of Combinatorial Theory, Series B,
Vol. 122,
Issue. ,
p.
578.
Fife, Tara
and
Oxley, James
2017.
Laminar matroids.
European Journal of Combinatorics,
Vol. 62,
Issue. ,
p.
206.
Fife, Tara
and
Oxley, James
2019.
Generalized laminar matroids.
European Journal of Combinatorics,
Vol. 79,
Issue. ,
p.
111.
Bonin, Joseph E.
and
Long, Kevin
2023.
The Excluded Minors for Three Classes of 2-Polymatroids Having Special Types of Natural Matroids.
SIAM Journal on Discrete Mathematics,
Vol. 37,
Issue. 3,
p.
1715.
Mo, Songbao
2023.
Ideal hierarchical secret sharing and lattice path matroids.
Designs, Codes and Cryptography,
Vol. 91,
Issue. 4,
p.
1335.
Bonin, Joseph E.
2024.
A characterization of positroids, with applications to amalgams and excluded minors.
European Journal of Combinatorics,
Vol. 122,
Issue. ,
p.
104040.
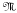 of matroids so obtained is closed under restriction, contraction, duality, truncation and elongation, but not under direct sum. We give an excluded-minor characterization of
of matroids so obtained is closed under restriction, contraction, duality, truncation and elongation, but not under direct sum. We give an excluded-minor characterization of 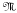 and show that
and show that 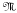 consists precisely of those transversal matroids with a presentation in which the sets in the presentation are nested. Finally, we show that on an n-set there are exactly 2n members of
consists precisely of those transversal matroids with a presentation in which the sets in the presentation are nested. Finally, we show that on an n-set there are exactly 2n members of 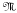 .
.