Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zhang, Daiqing
2018.
A Note on Marcinkiewicz Integrals along Submanifolds of Finite Type.
Journal of Function Spaces,
Vol. 2018,
Issue. ,
p.
1.
Yang, Yu-Guang
Li, Bo-Ran
Li, Dan
Zhou, Yi-Hua
and
Shi, Wei-Min
2019.
New quantum key agreement protocols based on Bell states.
Quantum Information Processing,
Vol. 18,
Issue. 10,
Li, Feng
Lei, Feng
Yanru, Cheng
Jun, Zhang
and
Sheng, Zhang
2019.
Trace level analysis and risk assessment of typical antibiotics in drinking water by liquid chromatography-tandem mass spectrometry (LC-MC/MS).
Desalination and Water Treatment,
Vol. 168,
Issue. ,
p.
184.
Yang, Yu-Guang
Li, Bo-Ran
Kang, Shuang-Yong
Chen, Xiu-Bo
Zhou, Yi-Hua
and
Shi, Wei-Min
2019.
New quantum key agreement protocols based on cluster states.
Quantum Information Processing,
Vol. 18,
Issue. 3,
Cao, Wei-Feng
and
Yang, Yu-Guang
2019.
Verifiable Quantum Secret Sharing Protocols Based on Four-Qubit Entangled States.
International Journal of Theoretical Physics,
Vol. 58,
Issue. 4,
p.
1202.
Yang, Yu-Guang
Gao, Shang
Zhou, Yi-Hua
and
Shi, Wei-Min
2019.
New Secure Quantum Dialogue Protocols over Collective Noisy Channels.
International Journal of Theoretical Physics,
Vol. 58,
Issue. 9,
p.
2810.
Yang, Yu-Guang
Bi, Jing-Lin
Li, Dan
Zhou, Yi-Hua
and
Shi, Wei-Min
2019.
Hash Function Based on Quantum Walks.
International Journal of Theoretical Physics,
Vol. 58,
Issue. 6,
p.
1861.
Yang, Yu-Guang
Cao, Sheng-Nan
Cao, Wei-Feng
Li, Dan
Zhou, Yi-Hua
and
Shi, Wei-Min
2019.
Generalized teleportation by means of discrete-time quantum walks on N-lines and N-cycles.
Modern Physics Letters B,
Vol. 33,
Issue. 06,
p.
1950070.
Xie, Meihua
Li, Haiyan
and
Zhao, Yuanjun
2020.
Blockchain financial investment based on deep learning network algorithm.
Journal of Computational and Applied Mathematics,
Vol. 372,
Issue. ,
p.
112723.
Liu, Weiming
and
Chen, Mu-Yen
2022.
Constructing a Music Network Teaching System by Using Neural Network Model with Wireless Audio Transmission.
Wireless Communications and Mobile Computing,
Vol. 2022,
Issue. ,
p.
1.
Al-Qassem, Hussain
and
Ali, Mohammed
2025.
A Note on Generalized Parabolic Marcinkiewicz Integrals with Grafakos–Stefanov Kernels.
Symmetry,
Vol. 17,
Issue. 3,
p.
429.
Ali, Mohammed
and
Al-Qassem, Hussain
2025.
On Rough Parametric Marcinkiewicz Integrals Along Certain Surfaces.
Mathematics,
Vol. 13,
Issue. 8,
p.
1287.
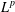 $L^{p}$ BOUNDS FOR NONISOTROPIC MARCINKIEWICZ INTEGRALS ASSOCIATED TO SURFACES
$L^{p}$ BOUNDS FOR NONISOTROPIC MARCINKIEWICZ INTEGRALS ASSOCIATED TO SURFACES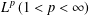 $L^{p}\,(1<p<\infty )$ estimates for nonisotropic Marcinkiewicz operators associated to surfaces under the integral kernels given by the elliptic sphere functions
$L^{p}\,(1<p<\infty )$ estimates for nonisotropic Marcinkiewicz operators associated to surfaces under the integral kernels given by the elliptic sphere functions 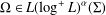 ${\rm\Omega}\in L(\log ^{+}L)^{{\it\alpha}}({\rm\Sigma})$ and the radial function
${\rm\Omega}\in L(\log ^{+}L)^{{\it\alpha}}({\rm\Sigma})$ and the radial function  $h\in {\mathcal{N}}_{{\it\beta}}(\mathbb{R}^{+})$. As applications, the corresponding results for parametric Marcinkiewicz integral operators related to area integrals and Littlewood–Paley
$h\in {\mathcal{N}}_{{\it\beta}}(\mathbb{R}^{+})$. As applications, the corresponding results for parametric Marcinkiewicz integral operators related to area integrals and Littlewood–Paley 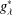 $g_{{\it\lambda}}^{\ast }$-functions are given.
$g_{{\it\lambda}}^{\ast }$-functions are given.