Article contents
UNDECIDABILITY OF THE FIRST ORDER THEORIES OF FREE NONCOMMUTATIVE LIE ALGEBRAS
Published online by Cambridge University Press: 23 October 2018
Abstract
Let R be a commutative integral unital domain and L a free noncommutative Lie algebra over R. In this article we show that the ring R and its action on L are 0-interpretable in L, viewed as a ring with the standard ring language 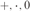 $+ , \cdot ,0$. Furthermore, if R has characteristic zero then we prove that the elementary theory
$+ , \cdot ,0$. Furthermore, if R has characteristic zero then we prove that the elementary theory 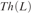 $Th\left( L \right)$ of L in the standard ring language is undecidable. To do so we show that the arithmetic
$Th\left( L \right)$ of L in the standard ring language is undecidable. To do so we show that the arithmetic  ${\Bbb N} = \langle {\Bbb N}, + , \cdot ,0\rangle $ is 0-interpretable in L. This implies that the theory of
${\Bbb N} = \langle {\Bbb N}, + , \cdot ,0\rangle $ is 0-interpretable in L. This implies that the theory of 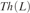 $Th\left( L \right)$ has the independence property. These results answer some old questions on model theory of free Lie algebras.
$Th\left( L \right)$ has the independence property. These results answer some old questions on model theory of free Lie algebras.
Keywords
Information
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2018
References
REFERENCES
- 1
- Cited by

