Hostname: page-component-745bb68f8f-b95js Total loading time: 0 Render date: 2025-01-12T02:52:14.611Z Has data issue: false hasContentIssue false
  • English
  • Français

A TOPOMETRIC EFFROS THEOREM

Part of: Topological and differentiable algebraic systems

Published online by Cambridge University Press:  02 February 2023

ITAÏ BEN YAACOV  and
JULIEN MELLERAY
ITAÏ BEN YAACOV*
Affiliation:
INSTITUT CAMILLE JORDAN CNRS UMR 5208 UNIVERSITÉ CLAUDE BERNARD—LYON 1 43 BOULEVARD DU 11 NOVEMBRE 1918 69622 VILLEURBANNE, FRANCE E-mail: melleray@math.univ-lyon1.fr URL: http://math.univ-lyon1.fr/~begnac/ URL: http://math.univ-lyon1.fr/~melleray/
JULIEN MELLERAY
Affiliation:
INSTITUT CAMILLE JORDAN CNRS UMR 5208 UNIVERSITÉ CLAUDE BERNARD—LYON 1 43 BOULEVARD DU 11 NOVEMBRE 1918 69622 VILLEURBANNE, FRANCE E-mail: melleray@math.univ-lyon1.fr URL: http://math.univ-lyon1.fr/~begnac/ URL: http://math.univ-lyon1.fr/~melleray/
*
E-mail: dontpublishmyemail@free.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

Given a continuous and isometric action of a Polish group G on an adequate Polish topometric space 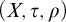$(X,\tau ,\rho )$ and 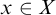$x \in X$, we find a necessary and sufficient condition for 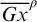$\overline {Gx}^{\rho }$ to be co-meagre; we also obtain a criterion that characterizes when such a point exists. This work completes a criterion established in earlier work of the authors.

Keywords

Polish grouptopometric spaceEffros theorem

MSC classification

Primary: 22A05: Structure of general topological groups
Type
Article
Information
The Journal of Symbolic Logic , First View , pp. 1 - 11
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ben Yaacov, I., Topometric spaces and perturbations of metric structures . Logic and Analysis , vol. 1 (2008), nos. 3–4, 235–272.CrossRefGoogle Scholar
Ben Yaacov, I., Berenstein, A., and Melleray, J., Polish topometric groups . Transactions of the American Mathematical Society , vol. 365 (2013), no. 7, pp. 3877–3897.CrossRefGoogle Scholar
Ben Yaacov, I. and Melleray, J., Grey subsets of Polish spaces, this Journal, vol. 80 (2015), no. 4, pp. 1379–1397.Google Scholar
Berenstein, A., Henson, C. W., and Ibarlucía, T., Existentially closed measure-preserving actions of free groups, preprint, 2022, arXiv:2203.10178.Google Scholar
Gao, S., Invariant Descriptive Set Theory , Pure and Applied Mathematics (Boca Raton), CRC Press, Boca Raton, 2009.Google Scholar