Article contents
Strong negative partition above the continuum
Published online by Cambridge University Press: 12 March 2014
Extract
For e.g. λ = μ+, μ regular, λ larger than the continuum, we prove a strong nonpartition result (stronger than λ → [λ; λ]2). As a consequence, the product of two topological spaces of cellularity <λ may have cellularity λ, or, in equivalent formulation, the product of two λ-c.c. Boolean algebras may lack the λ-c.c. Also λ-S-spaces and λ-L-spaces exist. In fact we deal not with successors of regular λ but with regular λ above the continuum which has a nonreflecting stationary subset of ordinals with uncountable cofinalities; sometimes we require λ to be not strong limit.
The paper is self-contained. On the nonpartition results see the closely related papers of Todorčević [T1], Shelah [Sh276] and [Sh261], and Shelah and Steprans [ShSt1].
On the cellularity of products see Todorčević [T2] and [T3], where such results were obtained for (e.g.) cf  and
and 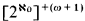 ; the class of cardinals he gets is quite disjoint from ours. In [Sh282] such results were obtained for more successors of singulars (mainly λ+, λ > 2cf λ). Also, concerning S and L spaces, Todorčević gets existence.
; the class of cardinals he gets is quite disjoint from ours. In [Sh282] such results were obtained for more successors of singulars (mainly λ+, λ > 2cf λ). Also, concerning S and L spaces, Todorčević gets existence.
Todorčević's work on cardinals like 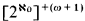 relies on [Sh68] (see more in [ShA2, Chapter XIII]) (the scales appearing in the proof of
relies on [Sh68] (see more in [ShA2, Chapter XIII]) (the scales appearing in the proof of 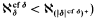 ). The problem was stressed in a preliminary version of the surveys of Juhasz and Monk. We give a detailed proof for one strong nonpartition theorem (1.1) and then give various strengthenings. We then use 1.10 to get the consequences (in 1.11 and 1.12).
). The problem was stressed in a preliminary version of the surveys of Juhasz and Monk. We give a detailed proof for one strong nonpartition theorem (1.1) and then give various strengthenings. We then use 1.10 to get the consequences (in 1.11 and 1.12).
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1990
References
REFERENCES
- 7
- Cited by

