Published online by Cambridge University Press: 12 March 2014
We are accustomed to regarding K as the weakest modal logic admitting of a relational semantics in the style made popular by Kripke. However, in a series of papers which demonstrates a startling connection between modal logic and the theory of paraconsistent inference, Ray Jennings and Peter Schotch have developed a generalized relational frame theory which articulates an infinite hierarchy of sublogics of K, each expressing a species of “weakly aggregative necessity”. Recall that K is axiomatized, in the presence of N and RM, by the schema of “binary aggregation”
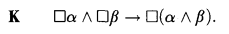
For each n ≥ 1, the weakly aggregative modal logic Kn is axiomatized by replacing K with the schema of “n-ary aggregation”

which is an n-ary relaxation, or weakening, of K. Note that K1 = K.
In [3], the authors claim without proof that Kn is determined by the class of frames F = (W, R), where W is a nonempty set and R is an (n + 1)-ary relation on W, under the generalization of Kriple's truth condition according to which □α is true at a point w in W if and only if α is true at one of x1,…,xn for all x1,…, xn in W such that Rw, x1,…, xn.