Hostname: page-component-cb9f654ff-plnhv Total loading time: 0.001 Render date: 2025-08-20T11:12:01.956Z Has data issue: false hasContentIssue false
  • English
  • Français

Solovay models and forcing extensions

Published online by Cambridge University Press:  12 March 2014

Joan Bagaria  and
Roger Bosch
Joan Bagaria
Affiliation:
Institució Catalana de Recerca i Estudis Avançats (Icrea), and Departament de Lògica, Història i Filosofia de la Ciència, Universitat de Barcelona, 08028 Barcelona, Catalonia, Spain, E-mail: bagaria@ub.edu
Roger Bosch
Affiliation:
Departamento de Filosofía, Universidad de Oviedo, 33071 Oviedo, Spain, E-mail: roger@pinon.ccu.uniovi.es
Get access Rights & Permissions [Opens in a new window]

Abstract.

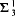We study the preservation under projective ccc forcing extensions of the property of L(ℝ) being a Solovay model. We prove that this property is preserved by every strongly- absolutely-ccc forcing extension, and that this is essentially the optimal preservation result, i.e., it does not hold for 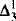absolutely-ccc forcing notions. We extend these results to the higher projective classes of ccc posets, and to the class of all projective ccc posets, using definably-Mahlo cardinals. As a consequence we obtain an exact equiconsistency result for generic absoluteness under projective absolutely-ccc forcing notions.

Keywords

Solovay modelsgeneric absolutenessdefinably-Mahlo cardinalsproductive-ccc partial orderings

Information

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 69 , Issue 3 , September 2004 , pp. 742 - 766
Copyright
Copyright © Association for Symbolic Logic 2004

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

REFERENCES

[1]Bagaria, J., Definable forcing and regularity properties of projective sets of reals, Ph.D. thesis, University of California at Berkeley, 1991.Google Scholar
[2]Bagaria, J. and Bosch, R., Projective forcing, Annals of Pure and Applied Logic, vol. 86 (1997), pp. 237–266.CrossRefGoogle Scholar
[3]Bagaria, J. and Bosch, R., Proper forcing extensions and Solovay models, to appear.Google Scholar
[4]Bagaria, J. and Friedman, S., Generic absoluteness, Annals of Pure and Applied Logic, vol. 108 (2001), pp. 3–13.CrossRefGoogle Scholar
[5]Bagaria, J. and Judah, H., Amoeba forcing, Suslin absoluteness and additivity of measure, Set theory of the continuum (Judah, H., Just, W., and Woodin, W. H., editors), MSRI, Berkeley, Springer-Verlag, Berlin, 1992.Google Scholar
[6]Jech, T., Set Theory, Springer Monographs in Mathematics, Springer-Verlag, Berlin, Heidelberg, 2003, The third millenium edition, revised and expanded.Google Scholar
[7]Jensen, R. B. and Solovay, R. M., Some applications of almost disjoint sets, Mathematical Logic and Foundations of Set Theory (Bar-Hillel, Y., editor), North Holland, Amsterdam, 1970.Google Scholar
[8]Judah, H. and Rosłanowski, A., Martin Axiom and the size of the continuum, this Journal, vol. 60 (1995), pp. 374–391.Google Scholar
[9]Judah, H. and Shelah, S., Souslin forcing, this Journal, vol. 53 (1988), pp. 1188–1207.Google Scholar
[10]Kanamori, A., The higher infinite: Large cardinals in set theory from their beginnings, Perspectives in Mathematical Logic, Springer-Verlag, Berlin, 1994.Google Scholar
[11]Shelah, S. and Woodin, W. H., Large cardinals imply that every reasonably definable set of reals is Lebesgue measurable, Israel Journal of Mathematics, vol. 70 (1990), no. 3, pp. 381–394.CrossRefGoogle Scholar
[12]Solovay, R., A model of set theory in which every set of reals is Lebesgue measurable, Annals of Mathematics, vol. 92 (1970), pp. 1–56.CrossRefGoogle Scholar
[13]Woodin, W. H., On the consistency strength of projective uniformization, Logic Colloquium'81 (Stern, J., editor), North-Holland, Amsterdam, 1982.Google Scholar