No CrossRef data available.
Published online by Cambridge University Press: 12 March 2014
The following fairly elementary result seems to raise possibilities for the study of countable models of a theory in a countable language. For the terminology of model theory we refer to [CK].
Let L be a countable first-order language. Let L′ be the relational language having, for each formula φ of L and each sequence υ1,…,υn of variables including the free variables of φ, an n-ary relation symbol Pφ. For any L-structure 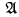 and any formula Ψ(υ) of L, we define the Ψ-fraction of
and any formula Ψ(υ) of L, we define the Ψ-fraction of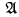 to be the L′-structure
to be the L′-structure 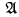 Ψ whose universe consists of those elements of
Ψ whose universe consists of those elements of 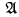 satisfying Ψ(υ) and whose relations {Rφ}φϵL are defined by letting a1,…,an satisfy Rφ in
satisfying Ψ(υ) and whose relations {Rφ}φϵL are defined by letting a1,…,an satisfy Rφ in 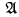 Ψ if, and only if, a1,…, an satisfy φ in
Ψ if, and only if, a1,…, an satisfy φ in 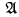 .
.
An L-elementary class means the class of all L-structures satisfying each of some set of sentences of L. The countable part of an L-elementary class K means the class of all countable L-structures from K.
Theorem. Let K be an L-elementary class and let Ψ(υ) be a formula of L. Then the class of countable Ψ-fractions of structures in K is the countable part of some L′-elementary class.
Comment. By the downward Löwenheim-Skolem theorem, the countable Ψ-fractions of structures in K are the same as the Ψ-fractions of countable structures in K.
Proof. We give a set Σ′ of L′-sentences whose countable models are exactly the countable Ψ-fractions of structures in K.