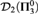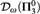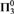Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Airey, Dylan
and
Mance, Bill
2017.
Normality of different orders for Cantor series expansions.
Nonlinearity,
Vol. 30,
Issue. 10,
p.
3719.
Airey, Dylan
Jackson, Steve
Kwietniak, Dominik
and
Mance, Bill
2020.
Borel complexity of sets of normal numbers via generic points in subshifts with specification.
Transactions of the American Mathematical Society,
Vol. 373,
Issue. 7,
p.
4561.
Airey, Dylan
Jackson, Steve
and
Mance, Bill
2022.
Some complexity results in the theory of normal numbers.
Canadian Journal of Mathematics,
Vol. 74,
Issue. 1,
p.
170.
DEKA, KONRAD
JACKSON, STEVE
KWIETNIAK, DOMINIK
and
MANCE, BILL
2025.
Borel complexity of sets of points with prescribed Birkhoff averages in Polish dynamical systems with a specification property.
Ergodic Theory and Dynamical Systems,
p.
1.