Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Toffalori, Carlo
1986.
Locally p‐ℵ0‐Categorical Theories.
Mathematical Logic Quarterly,
Vol. 32,
Issue. 19-24,
p.
341.
Marcja, Annalisa
Prest, Mike
and
Toffalori, Carlo
1991.
Classification theory for abelian groups with an endomorphism.
Archive for Mathematical Logic,
Vol. 31,
Issue. 2,
p.
95.
Lenski, Wolfgang
1995.
Quantifiers: Logics, Models and Computation.
p.
139.
Aschenbrenner, Matthias
Dolich, Alf
Haskell, Deirdre
Macpherson, Dugald
and
Starchenko, Sergei
2013.
Vapnik–Chervonenkis Density in Some Theories without the Independence Property, II.
Notre Dame Journal of Formal Logic,
Vol. 54,
Issue. 3-4,
Conant, Gabriel
2019.
Stability and sparsity in sets of natural numbers.
Israel Journal of Mathematics,
Vol. 230,
Issue. 1,
p.
471.


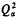 -core sentences and positive primitive formulas. For complete extensions of the elementary theory of R-modules it follows that all Ramsey quantifiers (ℵ
-core sentences and positive primitive formulas. For complete extensions of the elementary theory of R-modules it follows that all Ramsey quantifiers (ℵ