Article contents
Jonsson-like partition relations and j: V → V
Published online by Cambridge University Press: 12 March 2014
Abstract.
Working in the theory ”ZF + There is a nontrivial elementary embedding j : V → V“, we show that a final segment of cardinals satisfies certain square bracket finite and infinite exponent partition relations. As a corollary to this, we show that this final segment is composed of Jonsson cardinals. We then show how to force and bring this situation down to small alephs. A prototypical result is the construction of a model for ZF in which every cardinal μ ≥ ℵ2 satisfies the square bracket infinite exponent partition relation 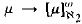 . We conclude with a discussion of some consistency questions concerning different versions of the axiom asserting the existence of a nontrivial elementary embedding j: V → V. By virtue of Kunen's celebrated inconsistency result, we use only a restricted amount of the Axiom of Choice.
. We conclude with a discussion of some consistency questions concerning different versions of the axiom asserting the existence of a nontrivial elementary embedding j: V → V. By virtue of Kunen's celebrated inconsistency result, we use only a restricted amount of the Axiom of Choice.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 2004
References
REFERENCES
- 1
- Cited by

