Published online by Cambridge University Press: 12 March 2014
Given an abstract logic 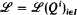 , generated by a set of quantifiers Qi, one can construct for each type τ a topological space Sτ, exactly as one constructs the Stone space for τ in first-order logic. Letting T be an arbitrary directed set of types, the set
, generated by a set of quantifiers Qi, one can construct for each type τ a topological space Sτ, exactly as one constructs the Stone space for τ in first-order logic. Letting T be an arbitrary directed set of types, the set 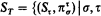
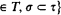 is an inverse topological system whose bonding mappings
is an inverse topological system whose bonding mappings  are naturally determined by the reduct operation on structures. We relate the compactness of
are naturally determined by the reduct operation on structures. We relate the compactness of 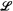 to the topological properties of ST. For example, if I is countable then
to the topological properties of ST. For example, if I is countable then 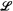 is compact iff for every τ each clopen subset of Sτ is of finite type and Sτ, is homeomorphic to limST, where T is the set of finite subtypes of τ. We finally apply our results to concrete logics.
is compact iff for every τ each clopen subset of Sτ is of finite type and Sτ, is homeomorphic to limST, where T is the set of finite subtypes of τ. We finally apply our results to concrete logics.