Article contents
Incompatible Ω-Complete Theories
Published online by Cambridge University Press: 12 March 2014
Abstract
In 1985 the second author showed that if there is a proper class of measurable Woodin cardinals and 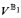 and
and 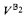 are generic extensions of V satisfying CH then
are generic extensions of V satisfying CH then 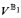 and
and 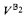 agree on all Σ12-statements. In terms of the strong logic Ω-logic this can be reformulated by saying that under the above large cardinal assumption ZFC + CH is Ω-complete for Σ12. Moreover, CH is the unique Σ12-statement with this feature in the sense that any other Σ12-statement with this feature is Ω-equivalent to CH over ZFC. It is natural to look for other strengthenings of ZFC that have an even greater degree of Ω-completeness. For example, one can ask for recursively enumerable axioms A such that relative to large cardinal axioms ZFC + A is Ω-complete for all of third-order arithmetic. Going further, for each specifiable segment Vλ of the universe of sets (for example, one might take Vλ to be the least level that satisfies there is a proper class of huge cardinals), one can ask for recursively enumerable axioms A such that relative to large cardinal axioms ZFC + A is Ω-complete for the theory of Vλ. If such theories exist, extend one another, and are unique in the sense that any other such theory B with the same level of Ω-completeness as A is actually Ω-equivalent to A over ZFC, then this would show that there is a unique Ω-complete picture of the successive fragments of the universe of sets and it would make for a very strong case for axioms complementing large cardinal axioms. In this paper we show that uniqueness must fail. In particular, we show that if there is one such theory that Ω-implies CH then there is another that Ω-implies ¬-CH.
agree on all Σ12-statements. In terms of the strong logic Ω-logic this can be reformulated by saying that under the above large cardinal assumption ZFC + CH is Ω-complete for Σ12. Moreover, CH is the unique Σ12-statement with this feature in the sense that any other Σ12-statement with this feature is Ω-equivalent to CH over ZFC. It is natural to look for other strengthenings of ZFC that have an even greater degree of Ω-completeness. For example, one can ask for recursively enumerable axioms A such that relative to large cardinal axioms ZFC + A is Ω-complete for all of third-order arithmetic. Going further, for each specifiable segment Vλ of the universe of sets (for example, one might take Vλ to be the least level that satisfies there is a proper class of huge cardinals), one can ask for recursively enumerable axioms A such that relative to large cardinal axioms ZFC + A is Ω-complete for the theory of Vλ. If such theories exist, extend one another, and are unique in the sense that any other such theory B with the same level of Ω-completeness as A is actually Ω-equivalent to A over ZFC, then this would show that there is a unique Ω-complete picture of the successive fragments of the universe of sets and it would make for a very strong case for axioms complementing large cardinal axioms. In this paper we show that uniqueness must fail. In particular, we show that if there is one such theory that Ω-implies CH then there is another that Ω-implies ¬-CH.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 2009
References
REFERENCES
- 12
- Cited by

