Published online by Cambridge University Press: 22 April 2015
Let Z2, Z3, and Z4 denote 2nd, 3rd, and 4th order arithmetic, respectively. We let Harrington’s Principle, HP, denote the statement that there is a real x such that every x-admissible ordinal is a cardinal in L. The known proofs of Harrington’s theorem “ $Det\left( {{\rm{\Sigma }}_1^1} \right)$ implies 0♯ exists” are done in two steps: first show that
$Det\left( {{\rm{\Sigma }}_1^1} \right)$ implies 0♯ exists” are done in two steps: first show that 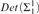 $Det\left( {{\rm{\Sigma }}_1^1} \right)$ implies HP, and then show that HP implies 0♯ exists. The first step is provable in Z2. In this paper we show that Z2 + HP is equiconsistent with ZFC and that Z3 + HP is equiconsistent with ZFC + there exists a remarkable cardinal. As a corollary, Z3 + HP does not imply 0♯ exists, whereas Z4 + HP does. We also study strengthenings of Harrington’s Principle over 2nd and 3rd order arithmetic.
$Det\left( {{\rm{\Sigma }}_1^1} \right)$ implies HP, and then show that HP implies 0♯ exists. The first step is provable in Z2. In this paper we show that Z2 + HP is equiconsistent with ZFC and that Z3 + HP is equiconsistent with ZFC + there exists a remarkable cardinal. As a corollary, Z3 + HP does not imply 0♯ exists, whereas Z4 + HP does. We also study strengthenings of Harrington’s Principle over 2nd and 3rd order arithmetic.