Article contents
Doughnuts, floating ordinals, square brackets, and ultraflitters
Published online by Cambridge University Press: 12 March 2014
Extract
In this paper, we study partition properties of the set of real numbers. The meaning of “set of real numbers” will vary, referring at times to the collection of sequences of natural numbers, ωω; the collection of infinite sets of natural numbers [ω]ω; the collection of infinite sequences of zeroes and ones, 2ω; or 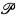 (ω), the power set of ω.
(ω), the power set of ω.
The archetype for the relations is the property: “all sets of reals are Ramsey,” in the notation of Erdős and Hajnal, ω → (ω)ω. This states that for every partition F : [ω]ω → 2, there is an infinite set H ∈ [ω]ω such that F is constant on [H]ω. Like virtually all of the properties we will discuss, it contradicts the Axiom of Choice but is compatible with the principle of dependent choices (DC). DC will be used throughout the paper wihtout further mention.
The properties discussed in this paper will vary in two respects. Some, like ω → (ω)ω, will be incompatible with the existence of an ultrafilter on ω (UF) and some will not. Some are known to be consistent relative to ZF alone, and for some, such as ω → (ω)ω, the question is still open. All properties, however, are true in Solovay's model and hence are consistent relative to Con(ZF + “there exists an inaccessible cardinal”).
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 2000
References
REFERENCES
- 7
- Cited by

