No CrossRef data available.
Published online by Cambridge University Press: 23 October 2018
This article presents a proof that Buss’s 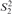 $S_2^2$ can prove the consistency of a fragment of Cook and Urquhart’s PV from which induction has been removed but substitution has been retained. This result improves Beckmann’s result, which proves the consistency of such a system without substitution in bounded arithmetic
$S_2^2$ can prove the consistency of a fragment of Cook and Urquhart’s PV from which induction has been removed but substitution has been retained. This result improves Beckmann’s result, which proves the consistency of such a system without substitution in bounded arithmetic 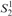 $S_2^1$.
$S_2^1$.
Our proof relies on the notion of “computation” of the terms of PV. In our work, we first prove that, in the system under consideration, if an equation is proved and either its left- or right-hand side is computed, then there is a corresponding computation for its right- or left-hand side, respectively. By carefully computing the bound of the size of the computation, the proof of this theorem inside a bounded arithmetic is obtained, from which the consistency of the system is readily proven.
This result apparently implies the separation of bounded arithmetic because Buss and Ignjatović stated that it is not possible to prove the consistency of a fragment of PV without induction but with substitution in Buss’s 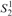 $S_2^1$. However, their proof actually shows that it is not possible to prove the consistency of the system, which is obtained by the addition of propositional logic and other axioms to a system such as ours. On the other hand, the system that we have considered is strictly equational, which is a property on which our proof relies.
$S_2^1$. However, their proof actually shows that it is not possible to prove the consistency of the system, which is obtained by the addition of propositional logic and other axioms to a system such as ours. On the other hand, the system that we have considered is strictly equational, which is a property on which our proof relies.