Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Seetapun, David
and
Slaman, Theodore A.
1995.
On the Strength of Ramsey's Theorem.
Notre Dame Journal of Formal Logic,
Vol. 36,
Issue. 4,
Avigad, Jeremy
1996.
Formalizing forcing arguments in subsystems of second-order arithmetic.
Annals of Pure and Applied Logic,
Vol. 82,
Issue. 2,
p.
165.
Humphreys, A.
and
Simpson, Stephen
1996.
Separable Banach space theory needs strong set existence axioms.
Transactions of the American Mathematical Society,
Vol. 348,
Issue. 10,
p.
4231.
Makowsky, Johann A.
1997.
1995 European Summer Meeting of the Association for Symbolic Logic.
Bulletin of Symbolic Logic,
Vol. 3,
Issue. 1,
p.
73.
Simpson, Stephen G.
Tanaka, Kazuyuki
and
Yamazaki, Takeshi
2002.
Some conservation results on weak König's lemma.
Annals of Pure and Applied Logic,
Vol. 118,
Issue. 1-2,
p.
87.
Avigad, Jeremy
2004.
Forcing in Proof Theory.
Bulletin of Symbolic Logic,
Vol. 10,
Issue. 3,
p.
305.
Conidis, Chris J.
2010.
A measure-theoretic proof of Turing incomparability.
Annals of Pure and Applied Logic,
Vol. 162,
Issue. 1,
p.
83.
Simpson, Stephen G.
2014.
Baire Categoricity and Σ10-Induction.
Notre Dame Journal of Formal Logic,
Vol. 55,
Issue. 1,
Kreuzer, Alexander P.
and
Yokoyama, Keita
2016.
On principles between ∑1- and ∑2-induction, and monotone enumerations.
Journal of Mathematical Logic,
Vol. 16,
Issue. 01,
p.
1650004.
Hoyrup, Mathieu
2016.
Pursuit of the Universal.
Vol. 9709,
Issue. ,
p.
115.
Fernandes, António M.
2017.
Reverse Mathematics 2001.
p.
164.
Brattka, Vasco
2017.
Logic Colloquium '02.
p.
130.
Humphreys, A. James
2017.
Reverse Mathematics 2001.
p.
244.
Arana, Andrew
2017.
Simplicity: Ideals of Practice in Mathematics and the Arts.
p.
205.
Brattka, Vasco
Hendtlass, Matthew
and
Kreuzer, Alexander P.
2018.
On the Uniform Computational Content of the Baire Category Theorem.
Notre Dame Journal of Formal Logic,
Vol. 59,
Issue. 4,
Nemoto, Takako
Brattka, Vasco
Dzhafarov, Damir D.
Marcone, Alberto
and
Pauly, Arno
2020.
A constructive proof of the dense existence of nowhere-differentiable functions in C [ 0 , 1 ].
Computability,
Vol. 9,
Issue. 3-4,
p.
315.
Sanders, Sam
2022.
Representations and the Foundations of Mathematics.
Notre Dame Journal of Formal Logic,
Vol. 63,
Issue. 1,
SHORE, RICHARD A.
2023.
ALMOST THEOREMS OF HYPERARITHMETIC ANALYSIS.
The Journal of Symbolic Logic,
Vol. 88,
Issue. 2,
p.
664.
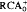 , and
, and  , show that
, show that 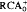 suffices to prove B.C.T.II. Some model theory of
suffices to prove B.C.T.II. Some model theory of  and its importance in view of Hilbert's program is discussed, as well as applications of our results to functional analysis.
and its importance in view of Hilbert's program is discussed, as well as applications of our results to functional analysis.