Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Blum, L.
Shub, M.
and
Smale, S.
1988.
On a theory of computation over the real numbers; NP completeness, recursive functions and universal machines.
p.
387.
Blum, Lenore
Shub, Mike
and
Smale, Steve
1989.
On a theory of computation and complexity over the real numbers: 𝑁𝑃- completeness, recursive functions and universal machines.
Bulletin of the American Mathematical Society,
Vol. 21,
Issue. 1,
p.
1.
Świerczkowski, S.
1990.
Interpretations of Euclidean geometry.
Transactions of the American Mathematical Society,
Vol. 322,
Issue. 1,
p.
315.
Lassez, Jean-Louis
1991.
Theoretical Aspects of Computer Software.
Vol. 526,
Issue. ,
p.
420.
Michaux, Christian
1991.
Ordered rings over which output sets are recursively enumerable sets.
Proceedings of the American Mathematical Society,
Vol. 112,
Issue. 2,
p.
569.
Byerly, Robert E.
1993.
Ordered subrings of the reals in which output sets are recursively enumerable.
Proceedings of the American Mathematical Society,
Vol. 118,
Issue. 2,
p.
597.
Campbell, L.A.
1994.
Rational Samuelson maps are univalent.
Journal of Pure and Applied Algebra,
Vol. 92,
Issue. 3,
p.
227.
Michaux, Christian
1994.
P≠NP over the nonstandard reals implies P≠NP over R.
Theoretical Computer Science,
Vol. 133,
Issue. 1,
p.
95.
Paredaens, J.
Van den Bussche, J.
and
Van Gucht, D.
1995.
First-order queries on finite structures over the reals.
p.
79.
Marker, David
1996.
Model Theory of Fields.
Vol. 5,
Issue. ,
p.
1.
Paredaens, Jan
Van den Bussche, Jan
and
Van Gucht, Dirk
1998.
First-Order Queries on Finite Structures Over the Reals.
SIAM Journal on Computing,
Vol. 27,
Issue. 6,
p.
1747.
Hemmerling, Armin
1998.
Computability of String Functions Over Algebraic Structures Armin Hemmerling.
Mathematical Logic Quarterly,
Vol. 44,
Issue. 1,
p.
1.
Baaz, Matthias
and
Veith, Helmut
1999.
Computer Science Logic.
Vol. 1584,
Issue. ,
p.
399.
Pheidas, Thanases
and
Zahidi, Karim
1999.
Undecidable existential theories of polynomial rings and function fields.
Communications in Algebra,
Vol. 27,
Issue. 10,
p.
4993.
Hemmerling, Armin
1999.
On approximate and algebraic computability over the real numbers.
Theoretical Computer Science,
Vol. 219,
Issue. 1-2,
p.
185.
Gyssens, Marc
Van den Bussche, Jan
and
Van Gucht, Dirk
1999.
Complete Geometric Query Languages.
Journal of Computer and System Sciences,
Vol. 58,
Issue. 3,
p.
483.
Hemmerling, Armin
2000.
On the Time Complexity of Partial Real Functions.
Journal of Complexity,
Vol. 16,
Issue. 1,
p.
363.
Michaux, C.
and
Troestler, C.
2000.
Isomorphism theorem for BSS recursively enumerable sets over real closed fields.
Theoretical Computer Science,
Vol. 231,
Issue. 2,
p.
253.
Van den Bussche, Jan
2000.
Constraint Databases.
p.
21.
Awodey, Steve
and
Reck, Erich H.
2002.
Completeness and Categoricity. Part I: Nineteenth-century Axiomatics to Twentieth-century Metalogic.
History and Philosophy of Logic,
Vol. 23,
Issue. 1,
p.
1.
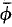 (X 1, …, Xm ) in
(X 1, …, Xm ) in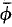 that uses only the axioms for real closed fields. (Reminder: real closed fields are ordered fields with the intermediate value property for polynomials.)
that uses only the axioms for real closed fields. (Reminder: real closed fields are ordered fields with the intermediate value property for polynomials.)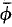 holds in all real closed fields rather than only in R; even the effectiveness of the passage from ϕ to
holds in all real closed fields rather than only in R; even the effectiveness of the passage from ϕ to 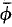 has found good theoretical uses besides firing the imagination.
has found good theoretical uses besides firing the imagination.