Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
BECKMANN, ARNOLD
and
BUSS, SAMUEL R.
2009.
POLYNOMIAL LOCAL SEARCH IN THE POLYNOMIAL HIERARCHY AND WITNESSING IN FRAGMENTS OF BOUNDED ARITHMETIC.
Journal of Mathematical Logic,
Vol. 09,
Issue. 01,
p.
103.
Krajíček, Jan
2010.
Computer Science Logic.
Vol. 6247,
Issue. ,
p.
22.
Thapen, Neil
2011.
Higher complexity search problems for bounded arithmetic and a formalized no-gap theorem.
Archive for Mathematical Logic,
Vol. 50,
Issue. 7-8,
p.
665.
Pollett, Chris
2011.
Conservative fragments of $${{S}^{1}_{2}}$$ and $${{R}^{1}_{2}}$$.
Archive for Mathematical Logic,
Vol. 50,
Issue. 3-4,
p.
367.
Kołodziejczyk, Leszek Aleksander
Nguyen, Phuong
and
Thapen, Neil
2011.
The provably total NP search problems of weak second order bounded arithmetic.
Annals of Pure and Applied Logic,
Vol. 162,
Issue. 6,
p.
419.
Pudlák, Pavel
and
Thapen, Neil
2012.
Alternating minima and maxima, Nash equilibria and Bounded Arithmetic.
Annals of Pure and Applied Logic,
Vol. 163,
Issue. 5,
p.
604.

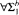 theorems of the fragments
theorems of the fragments 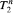 form a strictly increasing hierarchy. We shall show a link to some results about the lengths of proofs in predicate logic that supports the conjecture that the hierarchy is strictly increasing.
form a strictly increasing hierarchy. We shall show a link to some results about the lengths of proofs in predicate logic that supports the conjecture that the hierarchy is strictly increasing.