Hostname: page-component-7dd5485656-tbj44 Total loading time: 0 Render date: 2025-10-30T14:27:37.286Z Has data issue: false hasContentIssue false
  • English
  • Français

Complementation in the Turing degrees

Published online by Cambridge University Press:  12 March 2014

Theodore A. Slaman  and
John R. Steel
Theodore A. Slaman
Affiliation:
Department of Mathematics, University of Chicago, Chicago, Illinois 60637
John R. Steel
Affiliation:
Department of Mathematics, U. C. L. A., Los Angeles, California 90024
Get access Rights & Permissions [Opens in a new window]

Abstract

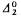Posner [6] has shown, by a nonuniform proof, that every degree has a complement below 0′. We show that a 1-generic complement for each 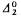set of degree between 0 and 0′ can be found uniformly. Moreover, the methods just as easily can be used to produce a complement whose jump has the degree of any real recursively enumerable in and above ∅′. In the second half of the paper, we show that the complementation of the degrees below 0′ does not extend to all recursively enumerable degrees. Namely, there is a pair of recursively enumerable degrees a above b such that no degree strictly below a joins b above a. (This result is independently due to S. B. Cooper.) We end with some open problems.

Information

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 54 , Issue 1 , March 1989 , pp. 160 - 176
Copyright
Copyright © Association for Symbolic Logic 1989

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

REFERENCES

Ά#x005B;1Ά#x005D;Cooper, S. B., The strong anticupping property for recursively enumerable degrees, this Journal (to appear).Google Scholar
Ά#x005B;2Ά#x005D;Jockusch, C. G. and Shore, R. A., REA operators, r. e. degrees and minimal covers, Recursion theory, Proceedings of Symposia in Pure Mathematics, vol. 42, American Mathematical Society, Providence, Rhode Island, 1985, pp. 3Ά#x2013;11.CrossRefGoogle Scholar
Ά#x005B;3Ά#x005D;Kleene, S. C. and Post, E. L., The upper semi-lattice of degrees of recursive unsolvability, Annals of Mathematics, ser. 2, vol. 59 (1954), pp. 379Ά#x2013;407.CrossRefGoogle Scholar
Ά#x005B;4Ά#x005D;Lachlan, A. H., The impossibility of finding relative complements for recursively enumerable degrees, this Journal, vol. 31 (1966), pp. 434Ά#x2013;454.Google Scholar
Ά#x005B;5Ά#x005D;Lachlan, A. H., Lower bounds for pairs of recursively enumerable degrees, Proceedings of the London Mathematical Society, ser. 3, vol. 16 (1966), pp. 537Ά#x2013;569.CrossRefGoogle Scholar
Ά#x005B;6Ά#x005D;Posner, D., The upper semilattice of degrees Ά#x2264; 0Ά#x2032; is complemented, this Journal, vol. 46 (1981), pp. 705Ά#x2013;713.Google Scholar
Ά#x005B;7Ά#x005D;Posner, D. and Robinson, R. W., Degrees joining to 0Ά#x2032;, this Journal, vol. 46 (1981), pp. 714Ά#x2013;722.Google Scholar
Ά#x005B;8Ά#x005D;Sacks, G. E., Degrees of unsolvability, rev. ed., Princeton University Press, Princeton, New Jersey, 1966.Google Scholar
Ά#x005B;9Ά#x005D;Shore, R. A., The theory of the degrees below 0Ά#x2032;, Journal of the London Mathematical Society, vol. 24 (1981), pp. 1Ά#x2013;14.CrossRefGoogle Scholar
Ά#x005B;10Ά#x005D;Slaman, T. A., A recursively enumerable degree that is not the top of a diamond (to appear).Google Scholar
Ά#x005B;11Ά#x005D;Spector, C., On degrees of recursive unsolvability, Annals of Mathematics, ser. 2, vol. 64 (1956), pp. 581Ά#x2013;592.CrossRefGoogle Scholar
Ά#x005B;12Ά#x005D;Yates, C. E. M., A minimal pair of recursively enumerable degrees, this Journal, vol. 31 (1966), pp. 159Ά#x2013;168.Google Scholar