Published online by Cambridge University Press: 01 August 2018
The shift map σ on ω* is the continuous self-map of ω* induced by the function n ↦ n + 1 on ω. Given a compact Hausdorff space X and a continuous function f : X → X, we say that (X, f) is a quotient of (ω*, σ) whenever there is a continuous surjection Q : ω*→ X such that Q ○ σ = σ ○ f.
Our main theorem states that if the weight of X is at most ℵ1, then (X, f) is a quotient of (ω*, σ), if and only if f is weakly incompressible (which means that no nontrivial open U ⊆ X has 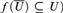 $f\left( {\bar{U}} \right) \subseteq U$). Under CH, this gives a complete characterization of the quotients of (ω*, σ) and implies, for example, that (ω*, σ−1) is a quotient of (ω*, σ).
$f\left( {\bar{U}} \right) \subseteq U$). Under CH, this gives a complete characterization of the quotients of (ω*, σ) and implies, for example, that (ω*, σ−1) is a quotient of (ω*, σ).
In the language of topological dynamics, our theorem states that a dynamical system of weight ℵ1 is an abstract ω-limit set if and only if it is weakly incompressible.
We complement these results by proving (1) our main theorem remains true when ℵ1 is replaced by any κ < p, (2) consistently, the theorem becomes false if we replace ℵ1 by ℵ2, and (3) OCA + MA implies that (ω*, σ−1) is not a quotient of (ω*, σ).