Article contents
Turbulent fluxes of entropy and internal energy in temperature stratified flows
Published online by Cambridge University Press: 03 August 2015
Abstract
We derive equations for the mean entropy and the mean internal energy in low-Mach-number temperature stratified turbulence (i.e. for turbulent convection or stably stratified turbulence), and show that turbulent flux of entropy is given by 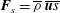 $\boldsymbol{F}_{s}=\overline{{\it\rho}}\,\overline{\boldsymbol{u}s}$ , where
$\boldsymbol{F}_{s}=\overline{{\it\rho}}\,\overline{\boldsymbol{u}s}$ , where 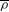 $\overline{{\it\rho}}$ is the mean fluid density,
$\overline{{\it\rho}}$ is the mean fluid density,  $s$ is fluctuation of entropy and overbars denote averaging over an ensemble of turbulent velocity fields,
$s$ is fluctuation of entropy and overbars denote averaging over an ensemble of turbulent velocity fields,  $\boldsymbol{u}$ . We demonstrate that the turbulent flux of entropy is different from the turbulent convective flux,
$\boldsymbol{u}$ . We demonstrate that the turbulent flux of entropy is different from the turbulent convective flux, 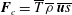 $\boldsymbol{F}_{c}=\overline{T}\,\overline{{\it\rho}}\,\overline{\boldsymbol{u}s}$ , of the fluid internal energy, where
$\boldsymbol{F}_{c}=\overline{T}\,\overline{{\it\rho}}\,\overline{\boldsymbol{u}s}$ , of the fluid internal energy, where 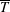 $\overline{T}$ is the mean fluid temperature. This turbulent convective flux is well-known in the astrophysical and geophysical literature, and it cannot be used as a turbulent flux in the equation for the mean entropy. This result is exact for low-Mach-number temperature stratified turbulence and is independent of the model used. We also derive equations for the velocity–entropy correlation,
$\overline{T}$ is the mean fluid temperature. This turbulent convective flux is well-known in the astrophysical and geophysical literature, and it cannot be used as a turbulent flux in the equation for the mean entropy. This result is exact for low-Mach-number temperature stratified turbulence and is independent of the model used. We also derive equations for the velocity–entropy correlation, 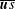 $\overline{\boldsymbol{u}s}$ , in the limits of small and large Péclet numbers, using the quasi-linear approach and the spectral
$\overline{\boldsymbol{u}s}$ , in the limits of small and large Péclet numbers, using the quasi-linear approach and the spectral  ${\it\tau}$ approximation, respectively. This study is important in view of different applications to astrophysical and geophysical temperature stratified turbulence.
${\it\tau}$ approximation, respectively. This study is important in view of different applications to astrophysical and geophysical temperature stratified turbulence.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press 2015
References
- 8
- Cited by

