Article contents
Stabilisation of short-wavelength instabilities by parallel-to-the-field shear in long-wavelength E × B flows
Published online by Cambridge University Press: 09 November 2020
Abstract
Magnetised plasma turbulence can have a multiscale character: instabilities driven by mean temperature gradients drive turbulence at the disparate scales of the ion and the electron gyroradii. Simulations of multiscale turbulence, using equations valid in the limit of infinite scale separation, reveal novel cross-scale interaction mechanisms in these plasmas. In the case that both long-wavelength (ion-gyroradius-scale) and short-wavelength (electron-gyroradius-scale) linear instabilities are driven far from marginal stability, we show that the short-wavelength instabilities are suppressed by interactions with long-wavelength turbulence. Two novel effects contributed to the suppression: parallel-to-the-field-line shearing by the long-wavelength 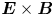 ${{\boldsymbol {E}} \times \boldsymbol {B}}$ flows, and the modification of the background density gradient by the piece of the long-wavelength electron adiabatic response with parallel-to-the-field-line variation. In contrast, simulations of multiscale turbulence where instabilities at both scales are driven near marginal stability demonstrate that when the long-wavelength turbulence is sufficiently collisional and zonally dominated the effect of cross-scale interaction can be parameterised solely in terms of the local modifications to the mean density and temperature gradients. We discuss physical arguments that qualitatively explain how a change in equilibrium drive leads to the observed transition in the impact of the cross-scale interactions.
${{\boldsymbol {E}} \times \boldsymbol {B}}$ flows, and the modification of the background density gradient by the piece of the long-wavelength electron adiabatic response with parallel-to-the-field-line variation. In contrast, simulations of multiscale turbulence where instabilities at both scales are driven near marginal stability demonstrate that when the long-wavelength turbulence is sufficiently collisional and zonally dominated the effect of cross-scale interaction can be parameterised solely in terms of the local modifications to the mean density and temperature gradients. We discuss physical arguments that qualitatively explain how a change in equilibrium drive leads to the observed transition in the impact of the cross-scale interactions.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © The Author(s), 2020. Published by Cambridge University Press
References
- 10
- Cited by


