No CrossRef data available.
Article contents
Non-diffusive angular momentum transport in rotating  $z$-pinches
$z$-pinches
Published online by Cambridge University Press: 04 November 2019
Abstract
The stability of conducting Taylor–Couette flows under the presence of toroidal magnetic background fields is considered. For strong enough magnetic amplitudes such magnetohydrodynamic flows are unstable against non-axisymmetric perturbations which may also transport angular momentum. In accordance with the often used diffusion approximation, one expects the angular momentum transport to be vanishing for rigid rotation. In the sense of a non-diffusive  $\unicode[STIX]{x1D6EC}$ effect, however, even for rigidly rotating
$\unicode[STIX]{x1D6EC}$ effect, however, even for rigidly rotating  $z$-pinches, an axisymmetric angular momentum flux appears which is directed outward (inward) for large (small) magnetic Mach numbers. The internal rotation in a magnetized rotating tank can thus never be uniform. Those particular rotation laws are used to estimate the value of the instability-induced eddy viscosity for which the non-diffusive
$z$-pinches, an axisymmetric angular momentum flux appears which is directed outward (inward) for large (small) magnetic Mach numbers. The internal rotation in a magnetized rotating tank can thus never be uniform. Those particular rotation laws are used to estimate the value of the instability-induced eddy viscosity for which the non-diffusive 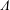 $\unicode[STIX]{x1D6EC}$ effect and the diffusive shear-induced transport compensate each other. The results provide the Shakura & Sunyaev viscosity ansatz leading to numerical values linearly growing with the applied magnetic field.
$\unicode[STIX]{x1D6EC}$ effect and the diffusive shear-induced transport compensate each other. The results provide the Shakura & Sunyaev viscosity ansatz leading to numerical values linearly growing with the applied magnetic field.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press 2019

