Article contents
Minimal perturbation flows that trigger mean field dynamos in shear flows
Published online by Cambridge University Press: 01 June 2018
Abstract
Using a variational optimization method we find the smallest flow perturbations that can trigger kinematic dynamo action in Kolmogorov flow. In comparison to previous work, a second-order mean field dynamo model is used to track down the optimal dynamos in the high magnetic Reynolds number limit (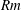 $Rm$ ). The magnitude of minimal perturbation flows decays inversely proportional to the magnetic Reynolds number. We reveal the asymptotic high-
$Rm$ ). The magnitude of minimal perturbation flows decays inversely proportional to the magnetic Reynolds number. We reveal the asymptotic high-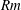 $Rm$ structure of the optimal flow perturbation and the magnetic eigenmode. We identify the optimal dynamo as of
$Rm$ structure of the optimal flow perturbation and the magnetic eigenmode. We identify the optimal dynamo as of  $\unicode[STIX]{x1D6FC}{-}\unicode[STIX]{x1D6FA}$ type, with magnetic fluctuations that localize on a critical layer.
$\unicode[STIX]{x1D6FC}{-}\unicode[STIX]{x1D6FA}$ type, with magnetic fluctuations that localize on a critical layer.
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press 2018
References
Ben-Dov, G. & Cohen, J.
2007
Critical Reynolds number for a natural transition to turbulence in pipe flows. Phys. Rev. Lett.
98, 064503.CrossRefGoogle ScholarPubMed
Biau, D. & Bottaro, A.
2009
An optimal path to transition in a duct. Phil. Trans. R. Soc. Lond. A
367 (1888), 529–544.Google Scholar
Braginsky, S.
1964
Self excitation of a magnetic field during the motion of a highly conducting fluid. Sov. Phys. JETP
20, 726–735.Google Scholar
Braginsky, S. & Roberts, P.
1975
Magnetic field generation by baroclinic waves. Proc. R. Soc. Lond. A
347 (1648), 125–140.Google Scholar
Chapman, S. J.
2002
Subcritical transition in channel flows. J. Fluid Mech.
451, 35–97.Google Scholar
Chen, L., Herreman, W. & Jackson, A.
2015
Optimal dynamo action by steady flows confined to a cube. J. Fluid Mech.
783, 23–45.Google Scholar
Chen, L., Herreman, W., Li, K., Livermore, P. W., Luo, J. W. & Jackson, A.
2018
The optimal kinematic dynamo driven by steady flows in a sphere. J. Fluid Mech.
839, 1–32.Google Scholar
Cherubini, S., Palma, P. D. & Robinet, J.-C.
2015
Nonlinear optimals in the asymptotic suction boundary layer: transition thresholds and symmetry breaking. Phys. Fluids
27 (3), 034108.Google Scholar
Deguchi, K. & Hall, P.
2015
Asymptotic descriptions of oblique coherent structures in shear flows. J. Fluid Mech.
782, 356–367.Google Scholar
Duguet, Y., Brandt, L. & Larsson, B. R. J.
2010
Towards minimal perturbations in transitional plane Couette flow. Phys. Rev. E
82 (2), 026316.Google Scholar
Guseva, A., Hollerbach, R., Willis, A. P. & Avila, M.
2017
Dynamo action in a quasi-Keplerian Taylor–Couette flow. Phys. Rev. Lett.
119, 164501.Google Scholar
Hall, P. & Sherwin, S.
2010
Streamwise vortices in shear flows: harbingers of transition and the skeleton of coherent structures. J. Fluid Mech.
661, 178–205.Google Scholar
Herault, J., Rincon, F., Cossu, C., Lesur, G., Ogilvie, G. I. & Longaretti, P.-Y.
2011
Periodic magnetorotational dynamo action as a prototype of nonlinear magnetic-field generation in shear flows. Phys. Rev. E
84, 036321.Google Scholar
Herreman, W.
2016
Minimal flow perturbations that trigger kinematic dynamo in shear flows. J. Fluid Mech.
795, R1.Google Scholar
Hof, B., Juel, A. & Mullin, T.
2003
Scaling of the turbulence transition threshold in a pipe. Phys. Rev. Lett.
91 (24), 244502.CrossRefGoogle Scholar
Kerswell, R.
2018
Nonlinear nonmodal stability theory. Annu. Rev. Fluid Mech.
50 (1), 319–345.Google Scholar
Krause, F. & Rädler, K.-H.
1980
Mean-field Magnetohydrodynamics and Dynamo. Akademié and Pergamon.Google Scholar
Mellibovsky, F. & Meseguer, A.
2007
Pipe flow transition threshold following localized impulsive perturbations. Phys. Fluids
19 (4), 044102.Google Scholar
Moffatt, H. K.
1978
Magnetic Field Generation in Electrically Conducting Fluids. Cambridge University Press.Google Scholar
Peixinho, J. & Mullin, T.
2007
Finite-amplitude thresholds for transition in pipe flow. J. Fluid Mech.
582, 169–178.CrossRefGoogle Scholar
Pralits, J., Bottaro, A. & Cherubini, S.
2015
Weakly nonlinear optimal perturbations. J. Fluid Mech.
785, 135–151.Google Scholar
Rincon, F., Ogilvie, G. I. & Proctor, M. R. E.
2007
Self-sustaining nonlinear dynamo process in Keplerian shear flows. Phys. Rev. Lett.
98 (25), 254502.Google Scholar
Rincon, F., Ogilvie, G. I., Proctor, M. R. E. & Cossu, C.
2008
Subcritical dynamos in shear flows. Astron. Nachr.
329 (7), 750–761.Google Scholar
Riols, A., Rincon, F., Cossu, C., Lesur, G., Longaretti, P.-Y., Ogilvie, G. I. & Herault, J.
2013
Global bifurcations to subcritical magnetorotational dynamo action in Keplerian shear flow. J. Fluid Mech.
731, 1–45.Google Scholar
Riols, A., Rincon, F., Cossu, C., Lesur, G., Ogilvie, G. I. & Longaretti, P.-Y.
2015
Dissipative effects on the sustainment of a magnetorotational dynamo in Keplerian shear flow. Astron. Astrophys.
575, A14.CrossRefGoogle Scholar
Waleffe, F.
1997
On a self-sustaining process in shear flows. Phys. Fluids
9 (4), 883–900.Google Scholar
Wang, J., Gibson, J. & Waleffe, F.
2007
Lower branch coherent states in shear flows: transition and control. Phys. Rev. Lett.
98 (20), 204501.CrossRefGoogle ScholarPubMed
Willis, A. P.
2012
Optimization of the magnetic dynamo. Phys. Rev. Lett.
109 (25), 251101.Google Scholar
Zel’dovich, Y. B.
1957
The magnetic field in the two-dimensional motion of a conducting turbulent fluid. Sov. Phys. JETP
4, 460–462.Google Scholar
- 3
- Cited by

