Article contents
Analysis of the isotropic and anisotropic Grad–Shafranov equation
Published online by Cambridge University Press: 28 September 2021
Abstract
Pressure anisotropy is a commonly observed phenomenon in tokamak plasmas, due to external heating methods such as neutral beam injection and ion-cyclotron resonance heating. Equilibrium models for tokamaks are constructed by solving the Grad–Shafranov equation; such models, however, do not account for pressure anisotropy since ideal magnetohydrodynamics assumes a scalar pressure. A modified Grad–Shafranov equation can be derived to include anisotropic pressure and toroidal flow by including drift-kinetic effects from the guiding-centre model of particle motion. In this work, we have studied the mathematical well-posedness of these two problems by showing the existence and uniqueness of solutions to the Grad–Shafranov equation both in the standard isotropic case and when including pressure anisotropy and toroidal flow. A new fixed-point approach is used to show the existence of solutions in the Sobolev space $H_0^1$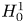 to the Grad–Shafranov equation, and sufficient criteria for their uniqueness are derived. The conditions required for the existence of solutions to the modified Grad–Shafranov equation are also constructed.
to the Grad–Shafranov equation, and sufficient criteria for their uniqueness are derived. The conditions required for the existence of solutions to the modified Grad–Shafranov equation are also constructed.
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 2
- Cited by


