Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zocco, A.
Aleynikova, K.
and
Xanthopoulos, P.
2018.
Strongly driven surface-global kinetic ballooning modes in general toroidal geometry.
Journal of Plasma Physics,
Vol. 84,
Issue. 3,
Ishizawa, A.
Imadera, K.
Nakamura, Y.
and
Kishimoto, Y.
2019.
Global gyrokinetic simulation of turbulence driven by kinetic ballooning mode.
Physics of Plasmas,
Vol. 26,
Issue. 8,
Cole, M. D. J.
Moritaka, T.
Hager, R.
Dominski, J.
Ku, S.
and
Chang, C. S.
2020.
Nonlinear global gyrokinetic delta-f turbulence simulations in a quasi-axisymmetric stellarator.
Physics of Plasmas,
Vol. 27,
Issue. 4,
Chowdhury, J
and
McMillan, B F
2021.
Low n electromagnetic modes in spherical tokamaks .
Plasma Physics and Controlled Fusion,
Vol. 63,
Issue. 7,
p.
075017.
Weir, G.M.
Xanthopoulos, P.
Hirsch, M.
Höfel, U.
Stange, T.
Pablant, N.
Grulke, O.
Äkäslompolo, S.
Alcusón, J.
Bozhenkov, S.
Beurskens, M.
Dinklage, A.
Fuchert, G.
Geiger, J.
Landreman, M.
Langenberg, A.
Lazerson, S.
Marushchenko, N.
Pasch, E.
Schilling, J.
Scott, E.R.
Turkin, Y.
and
Klinger, T.
2021.
Heat pulse propagation and anomalous electron heat transport measurements on the optimized stellarator W7-X.
Nuclear Fusion,
Vol. 61,
Issue. 5,
p.
056001.
McKinney, I.J.
Pueschel, M.J.
Faber, B.J.
Hegna, C.C.
Ishizawa, A.
and
Terry, P.W.
2021.
Kinetic-ballooning-mode turbulence in low-average-magnetic-shear equilibria.
Journal of Plasma Physics,
Vol. 87,
Issue. 3,
Helander, P.
and
Plunk, G.G.
2022.
Energetic bounds on gyrokinetic instabilities. Part 1. Fundamentals.
Journal of Plasma Physics,
Vol. 88,
Issue. 2,
Huang, Jie
Li, Mo-Shan
Qin, Cheng
and
Wang, Xian-Qu
2022.
Simulation of ion temperature gradient mode in Chinese First Quasi-axisymmetric Stellarator.
Acta Physica Sinica,
Vol. 71,
Issue. 18,
p.
185202.
Mishchenko, A
Bottino, A
Hayward-Schneider, T
Poli, E
Wang, X
Kleiber, R
Borchardt, M
Nührenberg, C
Biancalani, A
Könies, A
Lanti, E
Lauber, Ph
Hatzky, R
Vannini, F
Villard, L
and
Widmer, F
2022.
Gyrokinetic particle-in-cell simulations of electromagnetic turbulence in the presence of fast particles and global modes.
Plasma Physics and Controlled Fusion,
Vol. 64,
Issue. 10,
p.
104009.
Aleynikova, K.
Zocco, A.
and
Geiger, J.
2022.
Influence of magnetic configuration properties on kinetic ballooning modes in W7-X.
Journal of Plasma Physics,
Vol. 88,
Issue. 4,
Shen, Yong
Dong, J.Q.
Peng, X.D.
Han, M.K.
He, H.D.
and
Li, J.Q.
2022.
Extended instability of kinetic ballooning modes induced by ion temperature gradient and impurity in tokamaks.
Nuclear Fusion,
Vol. 62,
Issue. 10,
p.
106004.
Roberg-Clark, G. T.
Plunk, G. G.
Xanthopoulos, P.
Nührenberg, C.
Henneberg, S. A.
and
Smith, H. M.
2023.
Critical gradient turbulence optimization toward a compact stellarator reactor concept.
Physical Review Research,
Vol. 5,
Issue. 3,
Kennedy, D.
Giacomin, M.
Casson, F.J.
Dickinson, D.
Hornsby, W.A.
Patel, B.S.
and
Roach, C.M.
2023.
Electromagnetic gyrokinetic instabilities in STEP.
Nuclear Fusion,
Vol. 63,
Issue. 12,
p.
126061.
Mulholland, P.
Aleynikova, K.
Faber, B. J.
Pueschel, M. J.
Proll, J. H. E.
Hegna, C. C.
Terry, P. W.
and
Nührenberg, C.
2023.
Enhanced Transport at High Plasma Pressure and Subthreshold Kinetic Ballooning Modes in Wendelstein 7-X.
Physical Review Letters,
Vol. 131,
Issue. 18,
Mackenbach, R.J.J.
Proll, J.H.E.
Wakelkamp, R.
and
Helander, P.
2023.
The available energy of trapped electrons: a nonlinear measure for turbulent transport.
Journal of Plasma Physics,
Vol. 89,
Issue. 5,
Xu, J.Q.
Chen, W.
Peng, X.D.
Hao, G.Z.
Li, J.Q.
Qu, H.P.
Shi, P.W.
He, X.X.
Jiang, M.
and
Li, Y.G.
2023.
Gyrokinetic analysis of turbulent transport by electromagnetic turbulence in finite β plasmas with weak magnetic shear on HL-2A.
Nuclear Fusion,
Vol. 63,
Issue. 12,
p.
126031.
Shen, Yong
Dong, Jia-Qi
and
Li, Jia
2023.
Gyrokinetic Simulation of Kinetic Ballooning Mode and its Parametric Stabilization in Tokamak Plasmas With Impurities.
IEEE Transactions on Plasma Science,
Vol. 51,
Issue. 8,
p.
2134.
Mishchenko, Alexey
Borchardt, Matthias
Hatzky, Roman
Kleiber, Ralf
Könies, Axel
Nührenberg, Carolin
Xanthopoulos, Pavlos
Roberg-Clark, Gareth
and
Plunk, Gabriel G.
2023.
Global gyrokinetic simulations of electromagnetic turbulence in stellarator plasmas.
Journal of Plasma Physics,
Vol. 89,
Issue. 3,
Gaur, Rahul
Buller, Stefan
Ruth, Maximilian E.
Landreman, Matt
Abel, Ian G.
and
Dorland, William D.
2023.
An adjoint-based method for optimising MHD equilibria against the infinite-n, ideal ballooning mode.
Journal of Plasma Physics,
Vol. 89,
Issue. 5,
Gao, Yu
Geiger, Joachim
Jakubowski, Marcin W.
Pandey, Arun
Bozhenkov, Sergey
Feng, Yuhe
Endler, Michael
Baldzuhn, Jürgen
Perseo, Valeria
Kremeyer, Thierry
Schlisio, Georg
Puig Sitjes, Aleix
Otte, Matthias
Naujoks, Dirk
Krychowiak, Maciej
König, Ralf
Zhang, Daihong
Szepesi, Tamás
Kocsis, Gábor
Cseh, Gábor
Buzás, Attila
Pisano, Fabio
and
Knieps, Alexander
2024.
Heat and particle exhaust in high-performance plasmas in Wendelstein 7-X.
Nuclear Fusion,
Vol. 64,
Issue. 7,
p.
076060.
 $\unicode[STIX]{x1D6FD}$ plasmas, where
$\unicode[STIX]{x1D6FD}$ plasmas, where 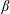 $\unicode[STIX]{x1D6FD}$ is the ratio of thermal to magnetic plasma pressure. The analysis shows suppression of ion-temperature-gradient (ITG) and trapped particle modes (TEM) by finite-
$\unicode[STIX]{x1D6FD}$ is the ratio of thermal to magnetic plasma pressure. The analysis shows suppression of ion-temperature-gradient (ITG) and trapped particle modes (TEM) by finite- $\unicode[STIX]{x1D6FD}$ effects and destabilization of KBMs at high
$\unicode[STIX]{x1D6FD}$ effects and destabilization of KBMs at high  $\unicode[STIX]{x1D6FD}$. The results are compared with a generic tokamak case. We show that, for large pressure gradients, the frequency of KBMs evaluated by the GENE code is in agreement with the analytical prediction of the diamagnetic modification of the ideal magnetohydrodynamic limit in W7-X general geometry. Thresholds for destabilization of the KBM are predicted for different W7-X equilibrium configurations. We discuss the relation of these thresholds to the ideal magnetohydrodynamic (MHD) stability properties of the corresponding equilibria.
$\unicode[STIX]{x1D6FD}$. The results are compared with a generic tokamak case. We show that, for large pressure gradients, the frequency of KBMs evaluated by the GENE code is in agreement with the analytical prediction of the diamagnetic modification of the ideal magnetohydrodynamic limit in W7-X general geometry. Thresholds for destabilization of the KBM are predicted for different W7-X equilibrium configurations. We discuss the relation of these thresholds to the ideal magnetohydrodynamic (MHD) stability properties of the corresponding equilibria.