Article contents
Theory of Endochronic Cyclic Viscoplasticity of Eutectic Tin/Lead Solder Alloy
Published online by Cambridge University Press: 05 May 2011
Abstract
In this paper, a theory of Endochronic cyclic viscoplasticity of eutectic Tin/Lead (60Sn/40Pb) solder alloy under cyclically thermomechanical strain histories had been established. Under the conditions of isotropic and inelastically incompressible small deformation, the constitutive equation of deviatoric behavior was expressed as:
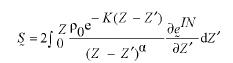
here  and the strain rate dependent intrinsic time scale
and the strain rate dependent intrinsic time scale 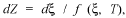
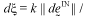
 and
and 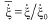 . Employing the experimental cyclic shear stress-strain curves of various testing temperature and frequency, all temp. dependent material parameters
. Employing the experimental cyclic shear stress-strain curves of various testing temperature and frequency, all temp. dependent material parameters  and
and 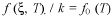 ; and the temp.-freq. dependent material function
; and the temp.-freq. dependent material function  were determined for temp. between 213K and 423K and freq. between 0.3Hz and 0.01Hz. Predicative capability of the theory were then challenged by a set of experiments with complicate strain history such as (i) Fast in tension/Slow in compression constant strain amplitude cyclic tests (ii) Slow-Fast-Slow constant amplitude cyclic tests. Through the excellent computational results, the present theory demonstrated that it can, not only play a vital role in the area of electronic solder mechanics, but also meet the needs of reliability analysis and life assessment in the electronic/photoelectronic packagings.
were determined for temp. between 213K and 423K and freq. between 0.3Hz and 0.01Hz. Predicative capability of the theory were then challenged by a set of experiments with complicate strain history such as (i) Fast in tension/Slow in compression constant strain amplitude cyclic tests (ii) Slow-Fast-Slow constant amplitude cyclic tests. Through the excellent computational results, the present theory demonstrated that it can, not only play a vital role in the area of electronic solder mechanics, but also meet the needs of reliability analysis and life assessment in the electronic/photoelectronic packagings.
Keywords
Information
- Type
- Articles
- Information
- Copyright
- Copyright © The Society of Theoretical and Applied Mechanics, R.O.C. 2006
References
- 8
- Cited by

