Article contents
A contribution to understanding of low-load spherical indentation—Comparison of tests on polymers and fused silica
Published online by Cambridge University Press: 13 September 2011
Abstract
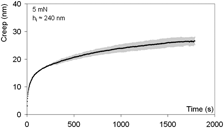
Spherical indentation, particularly at low loads, can ensure fully reversible deformations, suitable for the study of viscoelastic properties. However, there are growing concerns about the effective indenter radius in contact at small penetrations and the effect of thermal drift. The aim of this study was to characterize spherical indentation on polymethylmethacrylate (PMMA) and fused silica (FS). Several types of indentation experiments were performed on PMMA to determine its viscoelastic behavior, and a corresponding model was applied to calculate the main mechanical properties. A series of measurements on FS were performed to determine the effective indenter radius and thermal drift of the indentation system. It was shown that at low depths the effective radius of the spherical indenter can substantially differ from the nominal one and calibration of the indenter might be necessary for certain experiments. The effect of thermal drift and its consequences on creep measurements were discussed.
Information
- Type
- Articles
- Information
- Journal of Materials Research , Volume 27 , Issue 1: Focus Issue: Instrumented Indentation , 14 January 2012 , pp. 239 - 244
- Copyright
- Copyright © Materials Research Society 2011
References
REFERENCES
- 3
- Cited by

