No CrossRef data available.
Published online by Cambridge University Press: 15 March 2010
This paper is concerned with the theory of cup products in the Hopf cyclic cohomology of algebras and coalgebras. We show that the cyclic cohomology of a coalgebra can be obtained from a construction involving the noncommutative Weil algebra. Then we introduce the notion of higher 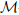 -twisted traces and use a generalization of the Quillen and Crainic constructions (see [14] and [3]) to define the cup product. We discuss the relation of the cup product above and S-operations on cyclic cohomology. We show that the product we define can be realized as a combination of the composition product in bivariant cyclic cohomology and a map from the cyclic cohomology of coalgebras to bivariant cohomology. In the last section, we briefly discuss the relation of our constructions with that in [9]. More precisely, we propose still another construction of such pairings which can be regarded as an intermediate step between the “Crainic” pairing and that of [9]. We show that it coincides with what in [9] and as far its relation to Crainic's construction is concerned, we reduce the question to a discussuion of a certain map in cohomology (see the question at the end of section 5).
-twisted traces and use a generalization of the Quillen and Crainic constructions (see [14] and [3]) to define the cup product. We discuss the relation of the cup product above and S-operations on cyclic cohomology. We show that the product we define can be realized as a combination of the composition product in bivariant cyclic cohomology and a map from the cyclic cohomology of coalgebras to bivariant cohomology. In the last section, we briefly discuss the relation of our constructions with that in [9]. More precisely, we propose still another construction of such pairings which can be regarded as an intermediate step between the “Crainic” pairing and that of [9]. We show that it coincides with what in [9] and as far its relation to Crainic's construction is concerned, we reduce the question to a discussuion of a certain map in cohomology (see the question at the end of section 5).
The results of the current paper were announced in [12].