Published online by Cambridge University Press: 07 November 2014
The theory of dendroidal sets has been developed to serve as a combinatorial model for homotopy coherent operads, see [MW07, CM13b]. An ∞-operad is a dendroidal set D satisfying certain lifting conditions.
In this paper we give a definition of K-groups Kn (D) for a dendroidal set D. These groups generalize the K-theory of symmetric monoidal (resp. permutative) categories and algebraic K-theory of rings. We establish some useful properties like invariance under the appropriate equivalences and long exact sequences which allow us to compute these groups in some examples. Using results from [Heu11b] and [BN12] we show that the K-theory groups of D can be realized as homotopy groups of a K-theory spectrum 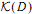 .
.