Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Aubert, Oriane
Le Bars, Michael
Le Gal, Patrice
and
Marcus, Philip S.
2012.
The universal aspect ratio of vortices in rotating stratified flows: experiments and observations.
Journal of Fluid Mechanics,
Vol. 706,
Issue. ,
p.
34.
Marcus, Philip S.
Pei, Suyang
Jiang, Chung-Hsiang
and
Hassanzadeh, Pedram
2013.
Three-Dimensional Vortices Generated by Self-Replication in Stably Stratified Rotating Shear Flows.
Physical Review Letters,
Vol. 111,
Issue. 8,
Aubert, O.
Le Bars, M.
Le Gal, P.
Marcus, P. S.
Barge, P.
and
Jorda, L.
2013.
Vortices in rotating and stratified flows: aspect ratio and sustainability.
EPJ Web of Conferences,
Vol. 46,
Issue. ,
p.
05004.
Marcus, Philip S.
Asay-Davis, Xylar
Wong, Michael H.
and
de Pater, Imke
2013.
Jupiter’s Red Oval BA: Dynamics, Color, and Relationship to Jovian Climate Change.
Journal of Heat Transfer,
Vol. 135,
Issue. 1,
Kurien, Susan
and
Smith, Leslie M.
2014.
Effect of rotation and domain aspect-ratio on layer formation in strongly stratified Boussinesq flows.
Journal of Turbulence,
Vol. 15,
Issue. 4,
p.
241.
Ungarish, Marius
2015.
On the coupling between spin-up and aspect ratio of vortices in rotating stratified flows: a predictive model.
Journal of Fluid Mechanics,
Vol. 777,
Issue. ,
p.
461.
Bashmachnikov, I.
Neves, F.
Calheiros, T.
and
Carton, X.
2015.
Properties and pathways of Mediterranean water eddies in the Atlantic.
Progress in Oceanography,
Vol. 137,
Issue. ,
p.
149.
Facchini, Giulio
and
Le Bars, Michael
2016.
On the lifetime of a pancake anticyclone in a rotating stratified flow.
Journal of Fluid Mechanics,
Vol. 804,
Issue. ,
p.
688.
Yim, Eunok
Billant, Paul
and
Ménesguen, Claire
2016.
Stability of an isolated pancake vortex in continuously stratified-rotating fluids.
Journal of Fluid Mechanics,
Vol. 801,
Issue. ,
p.
508.
De la Rosa Zambrano, H.M.
Cros, A.
Cruz Gómez, R.
Le Bars, M.
and
Le Gal, P.
2017.
A laboratory study of floating lenticular anticyclones.
European Journal of Mechanics - B/Fluids,
Vol. 61,
Issue. ,
p.
1.
Mahdinia, Mani
Hassanzadeh, Pedram
Marcus, Philip S.
and
Jiang, Chung-Hsiang
2017.
Stability of three-dimensional Gaussian vortices in an unbounded, rotating, vertically stratified, Boussinesq flow: linear analysis.
Journal of Fluid Mechanics,
Vol. 824,
Issue. ,
p.
97.
Gunn, K. L.
White, N. J.
Larter, R. D.
and
Caulfield, C. P.
2018.
Calibrated Seismic Imaging of Eddy‐Dominated Warm‐Water Transport Across the Bellingshausen Sea, Southern Ocean.
Journal of Geophysical Research: Oceans,
Vol. 123,
Issue. 4,
p.
3072.
Zhai, X. M.
and
Kurien, Susan
2018.
Characteristic length scales of strongly rotating Boussinesq flow in variable-aspect-ratio domains.
Journal of Fluid Mechanics,
Vol. 856,
Issue. ,
p.
397.
Khodkar, M. A.
Hassanzadeh, Pedram
Nabi, Saleh
and
Grover, Piyush
2019.
Reduced-order modeling of fully turbulent buoyancy-driven flows using the Green's function method.
Physical Review Fluids,
Vol. 4,
Issue. 1,
Srinivasan, Kaushik
McWilliams, James C.
Molemaker, M. Jeroen
and
Barkan, Roy
2019.
Submesoscale Vortical Wakes in the Lee of Topography.
Journal of Physical Oceanography,
Vol. 49,
Issue. 7,
p.
1949.
Galperin, Boris
and
Sukoriansky, Semion
2020.
Quasinormal scale elimination theory of the anisotropic energy spectra of atmospheric and oceanic turbulence.
Physical Review Fluids,
Vol. 5,
Issue. 6,
Couston, Louis-Alexandre
Lecoanet, Daniel
Favier, Benjamin
and
Le Bars, Michael
2020.
Shape and size of large-scale vortices: A generic fluid pattern in geophysical fluid dynamics.
Physical Review Research,
Vol. 2,
Issue. 2,
Orozco Estrada, A.
Cruz Gómez, Raúl C.
Cros, A.
and
Le Gal, P.
2020.
Coalescence of lenticular anticyclones in a linearly stratified rotating fluid.
Geophysical & Astrophysical Fluid Dynamics,
Vol. 114,
Issue. 4-5,
p.
504.
Lemasquerier, Daphné
Facchini, Giulio
Favier, Benjamin
and
Le Bars, Michael
2020.
Remote determination of the shape of Jupiter’s vortices from laboratory experiments.
Nature Physics,
Vol. 16,
Issue. 6,
p.
695.
Sutyrin, Georgi G.
2020.
How baroclinic vortices intensify resulting from erosion of their cores and/or changing environment.
Ocean Modelling,
Vol. 156,
Issue. ,
p.
101711.
 (vertical half-thickness over horizontal length scale) for steady and slowly evolving vortices in rotating stratified fluids, as a function of the Brunt–Väisälä frequencies within the vortex
(vertical half-thickness over horizontal length scale) for steady and slowly evolving vortices in rotating stratified fluids, as a function of the Brunt–Väisälä frequencies within the vortex 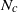 and in the background fluid outside the vortex
and in the background fluid outside the vortex 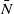 , the Coriolis parameter
, the Coriolis parameter  and the Rossby number
and the Rossby number 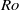 of the vortex:
of the vortex:  . This relation is valid for cyclones and anticyclones in either the cyclostrophic or geostrophic regimes; it works with vortices in Boussinesq fluids or ideal gases, and the background density gradient need not be uniform. Our relation for
. This relation is valid for cyclones and anticyclones in either the cyclostrophic or geostrophic regimes; it works with vortices in Boussinesq fluids or ideal gases, and the background density gradient need not be uniform. Our relation for  has many consequences for equilibrium vortices in rotating stratified flows. For example, cyclones must have
has many consequences for equilibrium vortices in rotating stratified flows. For example, cyclones must have 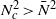 ; weak anticyclones (with
; weak anticyclones (with 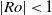 ) must have
) must have 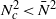 ; and strong anticyclones must have
; and strong anticyclones must have 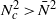 . We verify our relation for
. We verify our relation for  with numerical simulations of the three-dimensional Boussinesq equations for a wide variety of vortices, including: vortices that are initially in (dissipationless) equilibrium and then evolve due to an imposed weak viscous dissipation or density radiation; anticyclones created by the geostrophic adjustment of a patch of locally mixed density; cyclones created by fluid suction from a small localized region; vortices created from the remnants of the violent breakups of columnar vortices; and weakly non-axisymmetric vortices. The values of the aspect ratios of our numerically computed vortices validate our relationship for
with numerical simulations of the three-dimensional Boussinesq equations for a wide variety of vortices, including: vortices that are initially in (dissipationless) equilibrium and then evolve due to an imposed weak viscous dissipation or density radiation; anticyclones created by the geostrophic adjustment of a patch of locally mixed density; cyclones created by fluid suction from a small localized region; vortices created from the remnants of the violent breakups of columnar vortices; and weakly non-axisymmetric vortices. The values of the aspect ratios of our numerically computed vortices validate our relationship for  , and generally they differ significantly from the values obtained from the much-cited conjecture that
, and generally they differ significantly from the values obtained from the much-cited conjecture that 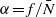 in quasi-geostrophic vortices.
in quasi-geostrophic vortices.
