Article contents
Two-dimensional numerical study of vortex shedding regimes of oscillatory flow past two circular cylinders in side-by-side and tandem arrangements at low Reynolds numbers
Published online by Cambridge University Press: 16 June 2014
Abstract
Oscillatory flow past two circular cylinders in side-by-side and tandem arrangements at low Reynolds numbers is simulated numerically by solving the two-dimensional Navier–Stokes (NS) equations using a finite-element method (FEM). The aim of this study is to identify the flow regimes of the two-cylinder system at different gap arrangements and Keulegan–Carpenter numbers (KC). Simulations are conducted at seven gap ratios  $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}G$ (
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}G$ ( 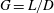 $G=L/D$ where
$G=L/D$ where 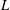 $L$ is the cylinder-to-cylinder gap and
$L$ is the cylinder-to-cylinder gap and  $D$ the diameter of a cylinder) of 0.5, 1, 1.5, 2, 3, 4 and 5 and KC ranging from 1 to 12 with an interval of 0.25. The flow regimes that have been identified for oscillatory flow around a single cylinder are also observed in the two-cylinder system but with different flow patterns due to the interactions between the two cylinders. In the side-by-side arrangement, the vortex shedding from the gap between the two cylinders dominates when the gap ratio is small, resulting in the gap vortex shedding (GVS) regime, which is different from any of the flow regimes identified for a single cylinder. For intermediate gap ratios of 1.5 and 2 in the side-by-side arrangement, the vortex shedding mode from one side of each cylinder is not necessarily the same as that from the other side, forming a so-called combined flow regime. When the gap ratio between the two cylinders is sufficiently large, the vortex shedding from each cylinder is similar to that of a single cylinder. In the tandem arrangement, when the gap between the two cylinders is very small, the flow regimes are similar to that of a single cylinder. For large gap ratios in the tandem arrangement, the vortex shedding flows from the gap side of the two cylinders interact and those from the outer sides of the cylinders are less affected by the existence of the other cylinder and similar to that of a single cylinder. Strong interaction between the vortex shedding flows from the two cylinders makes the flow very irregular at large KC values for both side-by-side and tandem arrangements.
$D$ the diameter of a cylinder) of 0.5, 1, 1.5, 2, 3, 4 and 5 and KC ranging from 1 to 12 with an interval of 0.25. The flow regimes that have been identified for oscillatory flow around a single cylinder are also observed in the two-cylinder system but with different flow patterns due to the interactions between the two cylinders. In the side-by-side arrangement, the vortex shedding from the gap between the two cylinders dominates when the gap ratio is small, resulting in the gap vortex shedding (GVS) regime, which is different from any of the flow regimes identified for a single cylinder. For intermediate gap ratios of 1.5 and 2 in the side-by-side arrangement, the vortex shedding mode from one side of each cylinder is not necessarily the same as that from the other side, forming a so-called combined flow regime. When the gap ratio between the two cylinders is sufficiently large, the vortex shedding from each cylinder is similar to that of a single cylinder. In the tandem arrangement, when the gap between the two cylinders is very small, the flow regimes are similar to that of a single cylinder. For large gap ratios in the tandem arrangement, the vortex shedding flows from the gap side of the two cylinders interact and those from the outer sides of the cylinders are less affected by the existence of the other cylinder and similar to that of a single cylinder. Strong interaction between the vortex shedding flows from the two cylinders makes the flow very irregular at large KC values for both side-by-side and tandem arrangements.
Information
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
References
- 48
- Cited by

